Vibrational Analysis of a (cutlery) Fork

I have published four papers and couple of webpages about the vibration and radiation of sound from tuning forks. A couple of months ago, I found a simple looking cutlery fork (the kind you use for eating) when I was cleaning out my research lab space and I was curious to see if a cutlery fork would exhibit any vibrational modes where the tines moved in opposite or alternating directions.

Modal Analysis

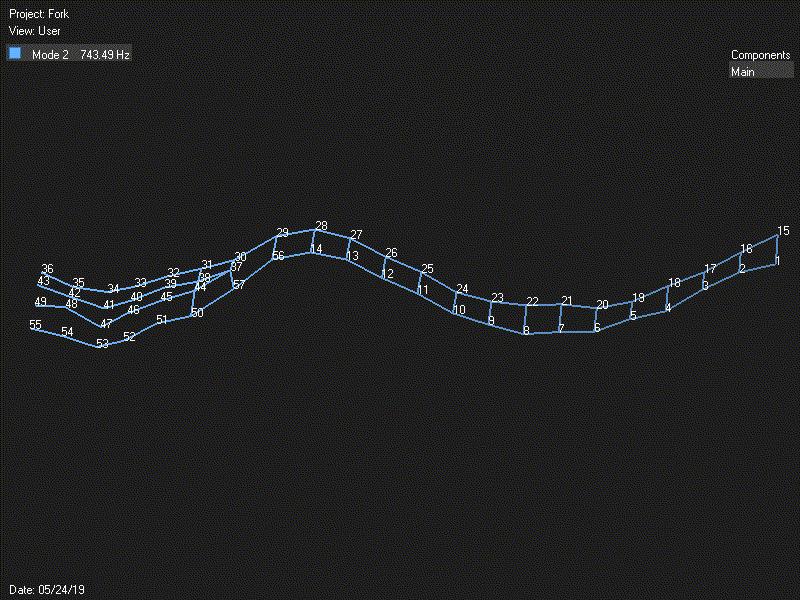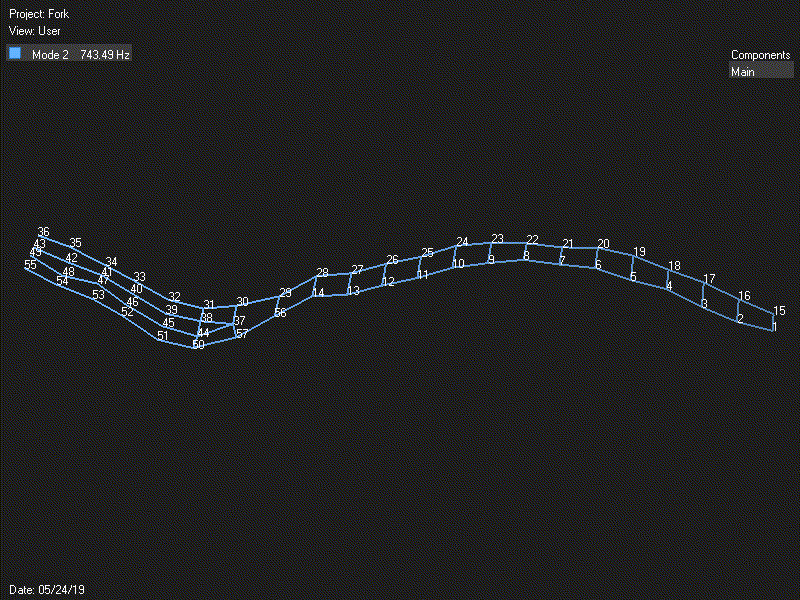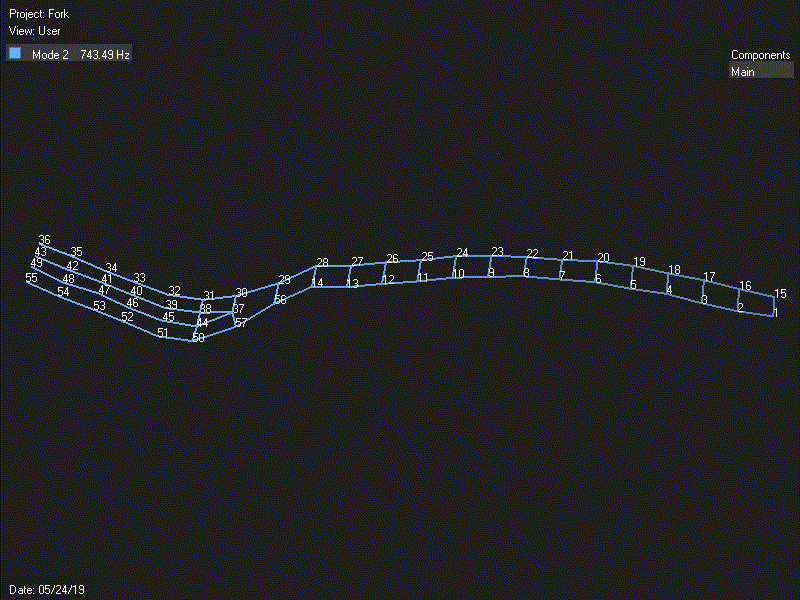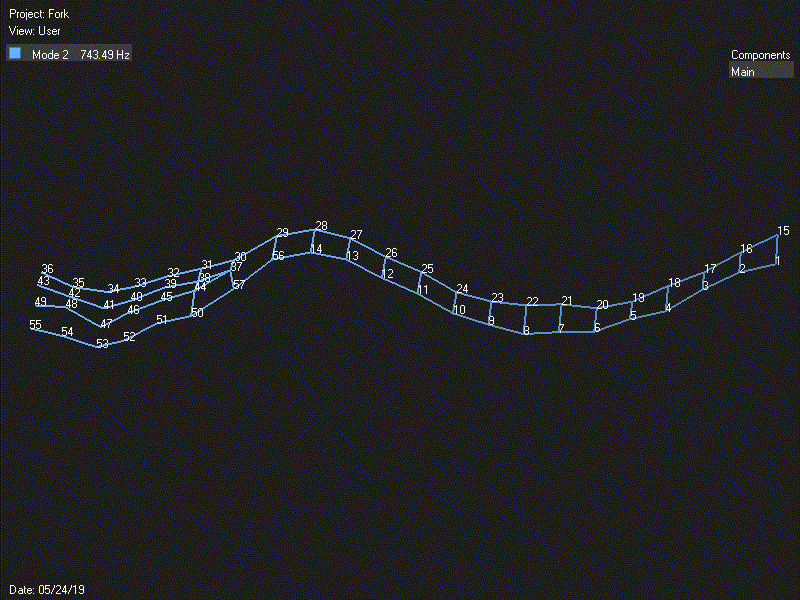
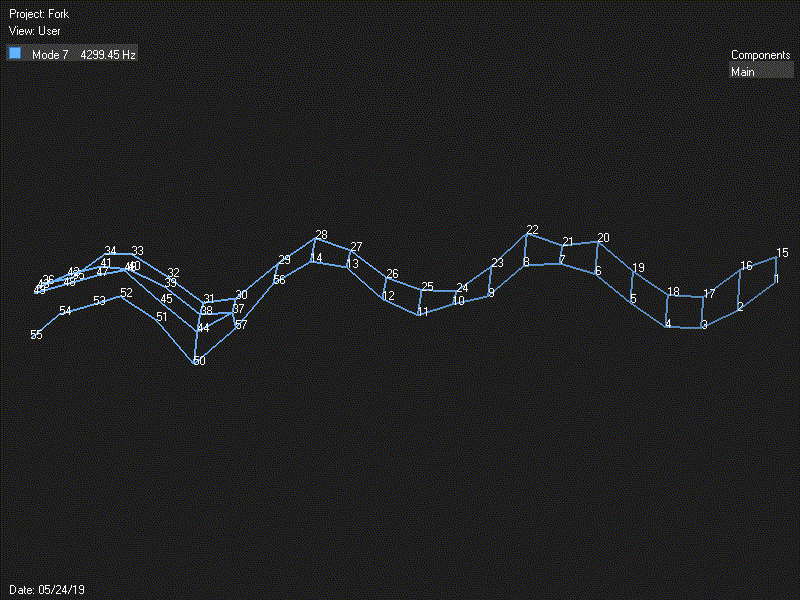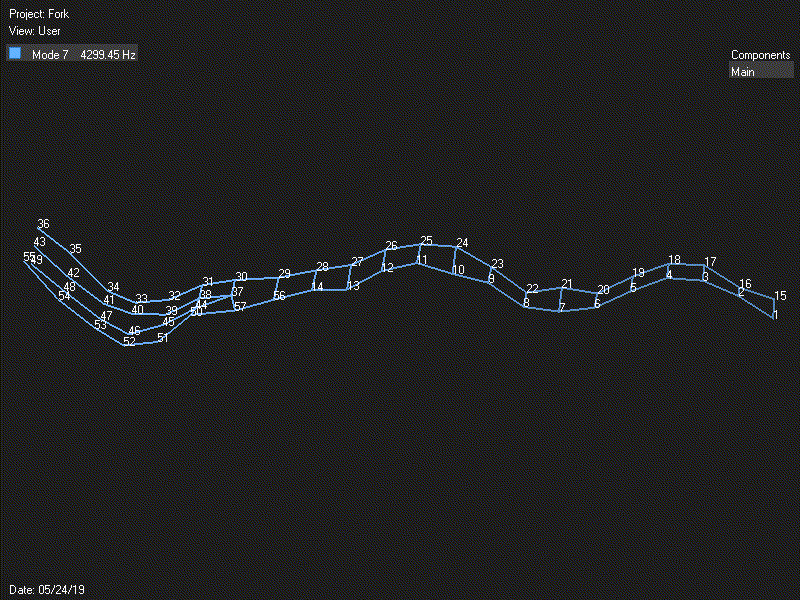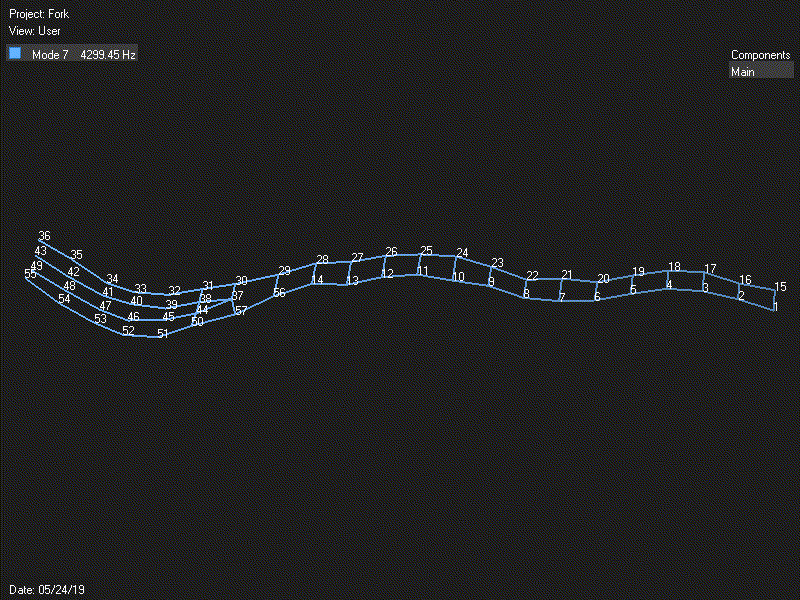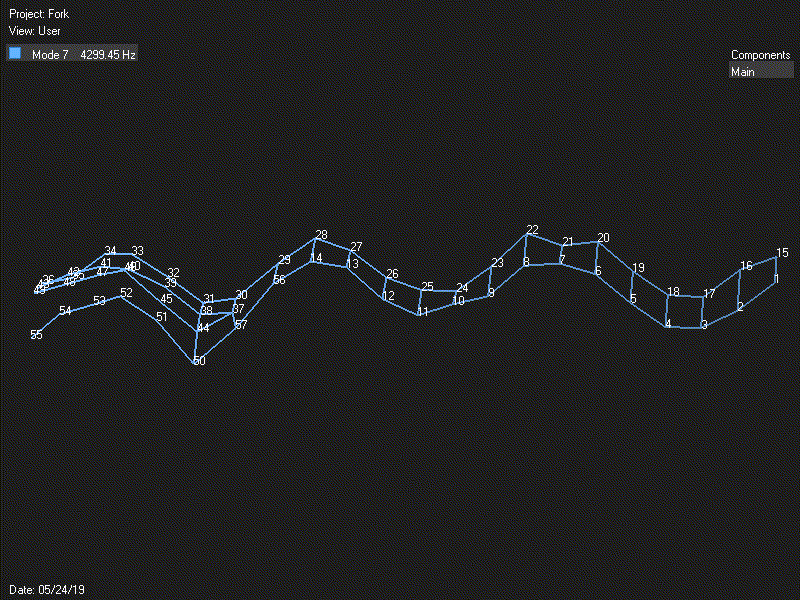
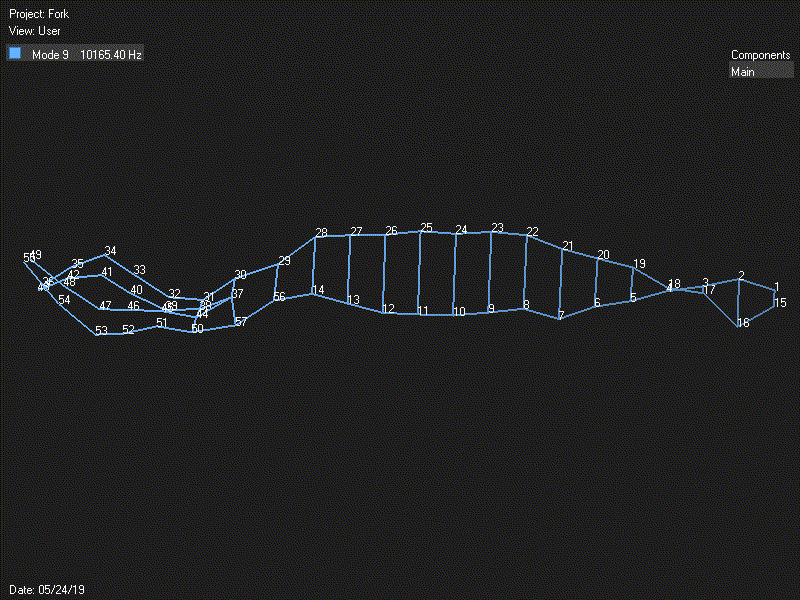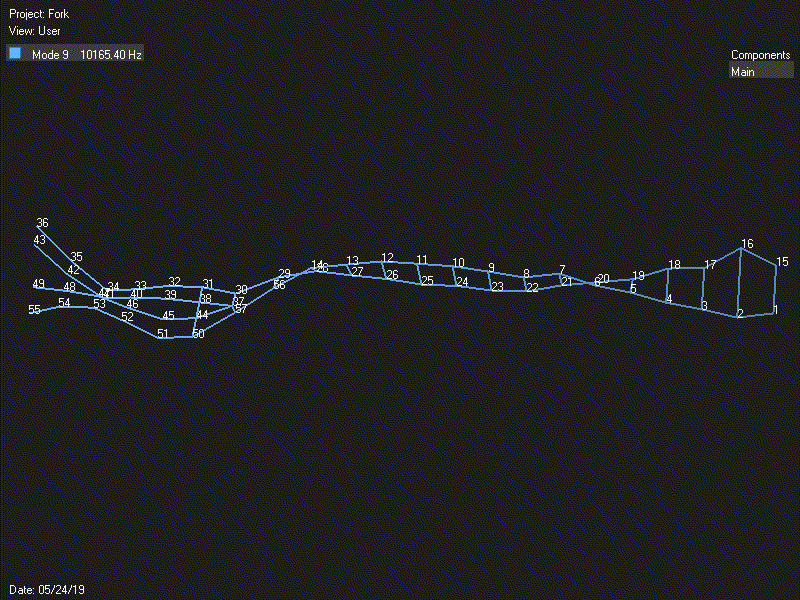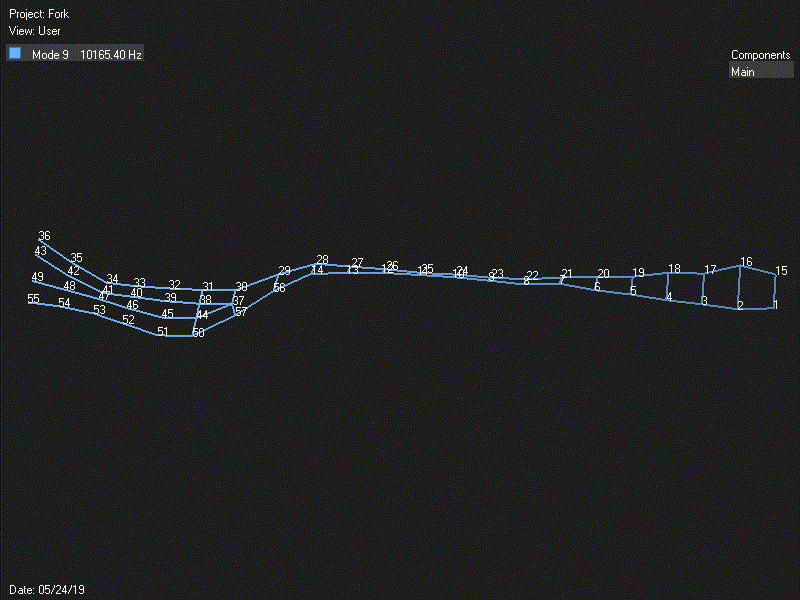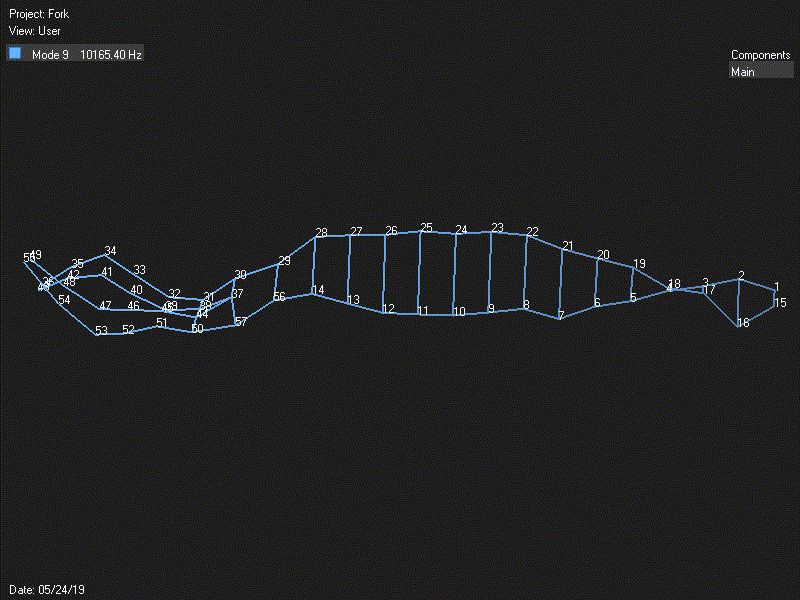
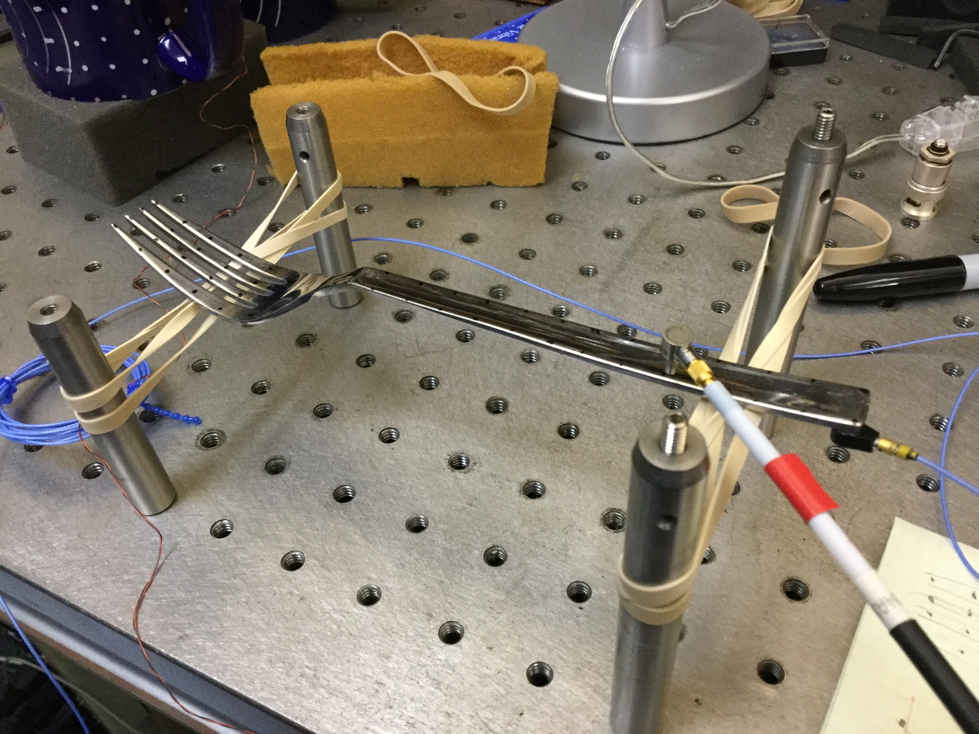
Experimental Modal Analysis is an experimental method that provides the vibrational mode shapes, their natural frequencies, and damping rates for a structure. A small (0.5g) accelerometer was attached underneath at the handle end of fork. A miniature impact force hammer was used to tap the fork at 1-cm intervals along the length of the fork. The ratio of acceleration to force (called the frequency response function) was recorded for each impact location. The collection of 57 frequency response functions was curve fit using STAR Modal software. The resulting relative amplitudes of motion corresponding to each measurement point were mapped to the grid point representation of the fork, resulting in shapes as shown further below.

photograph showing a cutlery fork suspensede by rubber bands at each end. A small accelerometer is attached to the underside of the handle at the end of the fork, and miniature impact hammer is shown resting on the rubber band supports. — The left photo shows the fork freely supported by rubber bands, with the accelerometer attached to the underside of the handle, and the miniature force hammer. The right photo shows the structure mesh grid in STAR Modal.

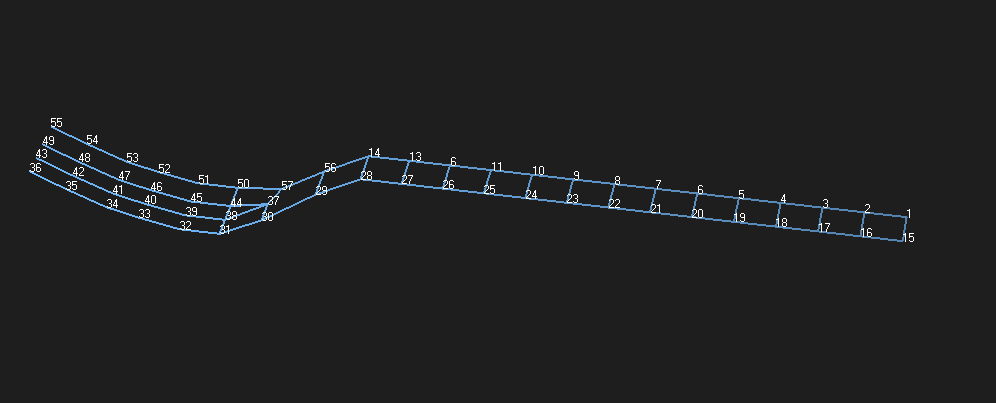
Grid points mapped to the shape of a cutlery fork. — The left photo shows the fork freely supported by rubber bands, with the accelerometer attached to the underside of the handle, and the miniature force hammer. The right photo shows the structure mesh grid in STAR Modal.

Mode Shapes

The resulting shapes weren't as exciting as I had hoped - and I was not able to observe any modes below 25,600 Hz where the tines moved in opposite directions (at least for a freely supported fork). I guess I shouldn't have been surprised to discover that for forks behaves pretty much like a slightly non-uniform free-free beam.

First vibrational mode of a fork (eating utensil). Two two ends of the fork move in the same direction while the middle of the fork moves in the opposite direction. — The mode on the left is the first bending mode at 269 Hz. The mode on the right is the second bending mode at 744 Hz.

Second vibrational mode of a fork (eating utensil). The mode shape is very similar to that of a free-free bar, with foru alternating regions of opposite direction movement. — The mode on the left is the first bending mode at 269 Hz. The mode on the right is the second bending mode at 744 Hz.

Torsional mode of a fork (eating utensil) in which the fork handle and tines twist along the long axis. — This second row shows the first torsional (twisting) mode at 1,940Hz on the left and the second torsional mode at 3,028 Hz on the right.