Acoustics and Vibration Animations Daniel A. Russell, Ph.D. Graduate Program in Acoustics The Pennsylvania State University All text and images on this page are ©2004-2011 by Daniel A. Russell and may not used in other web pages or reports without permission.

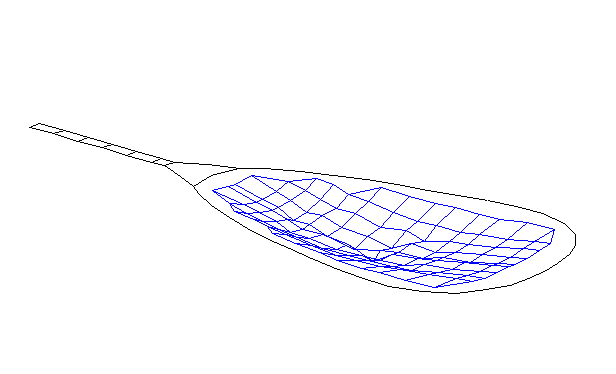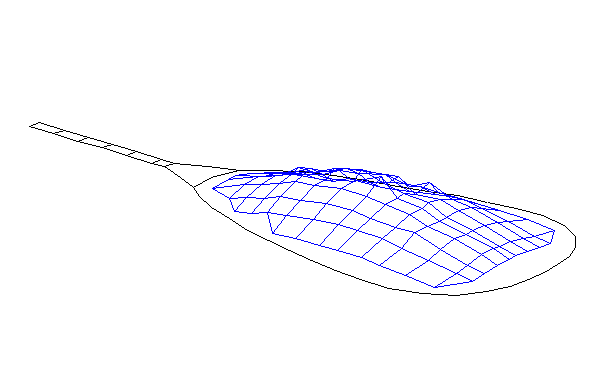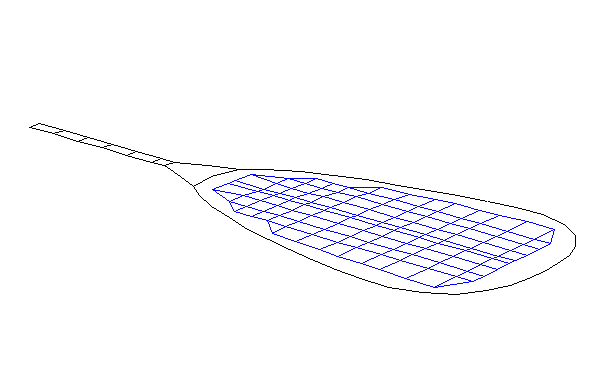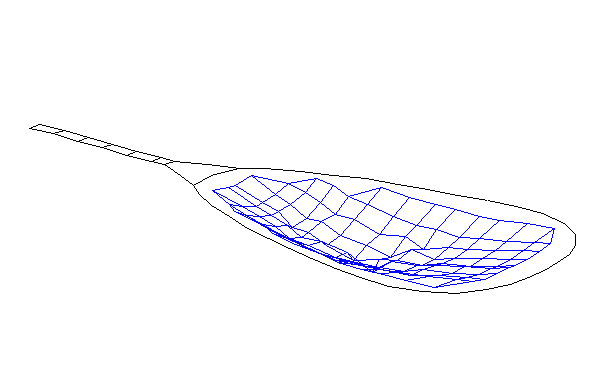
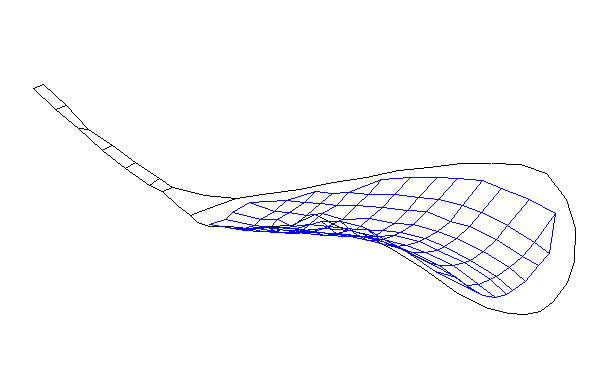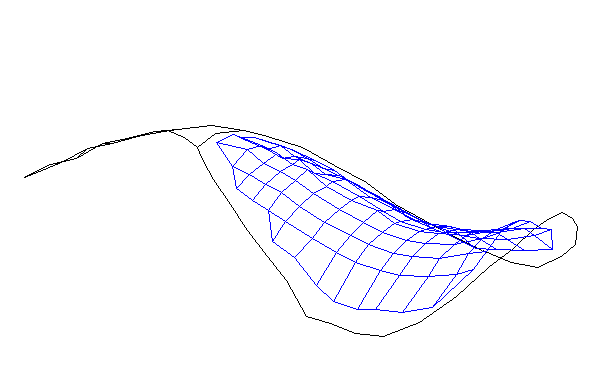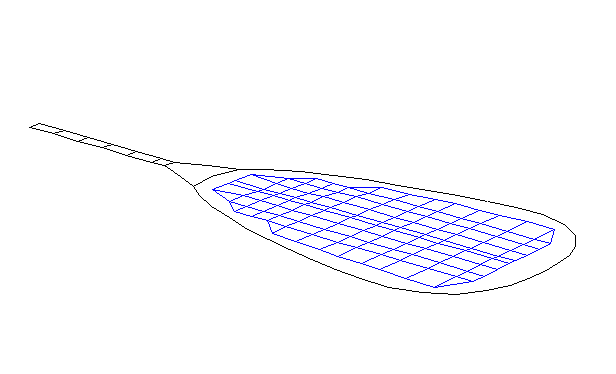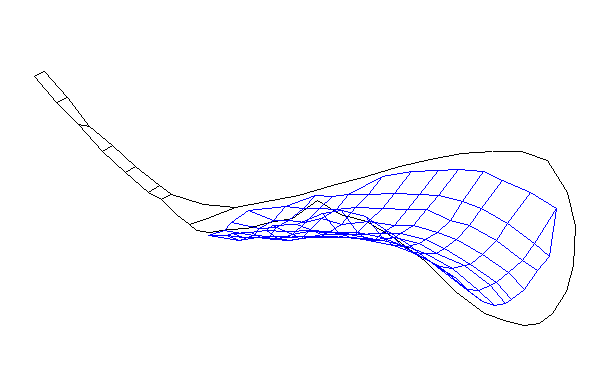
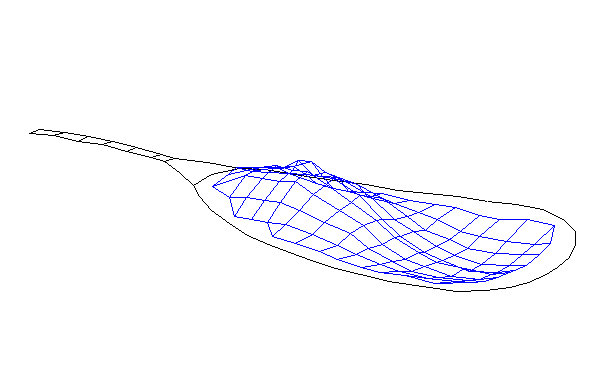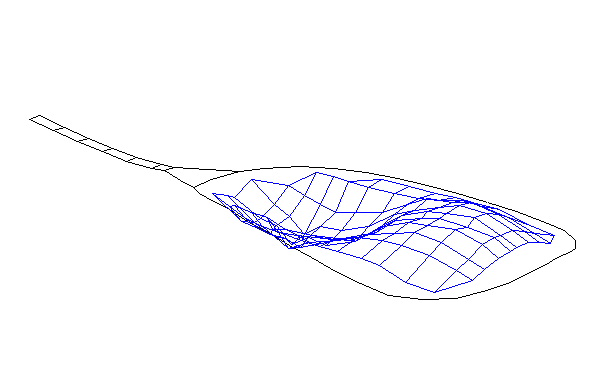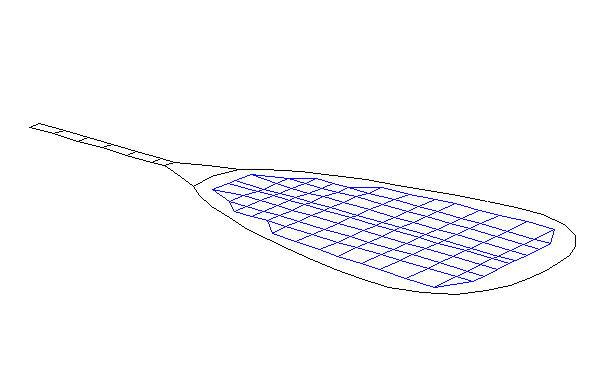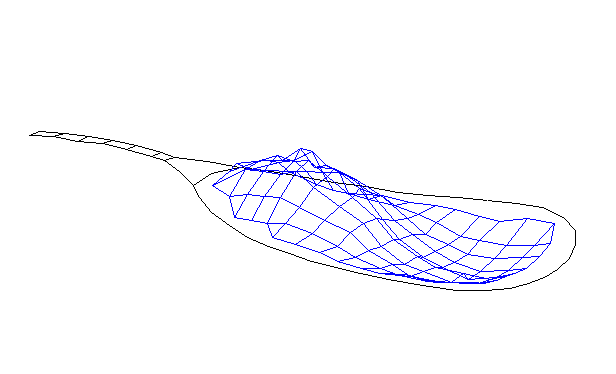
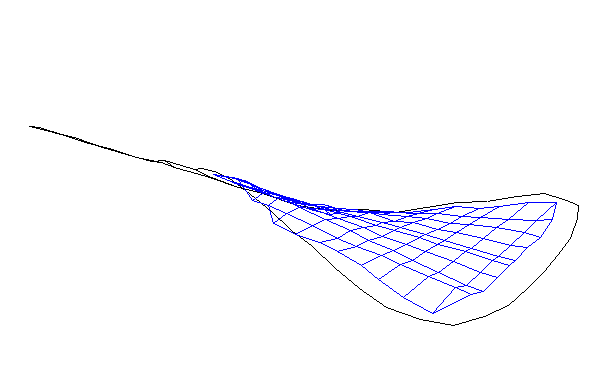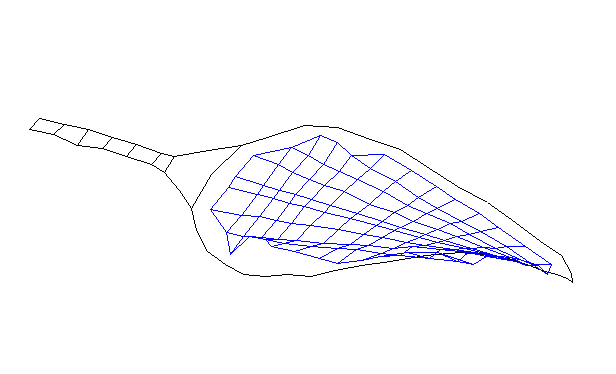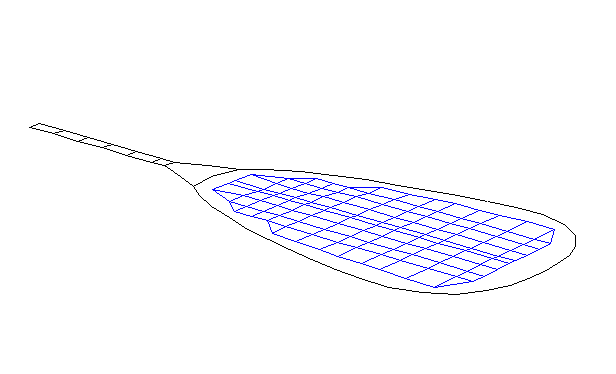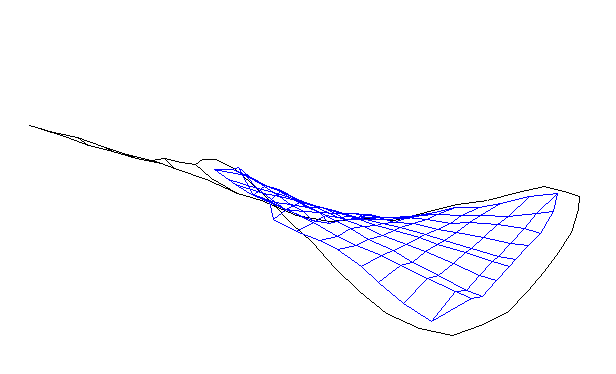
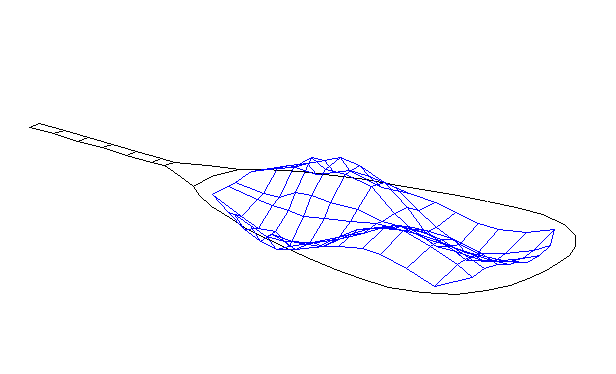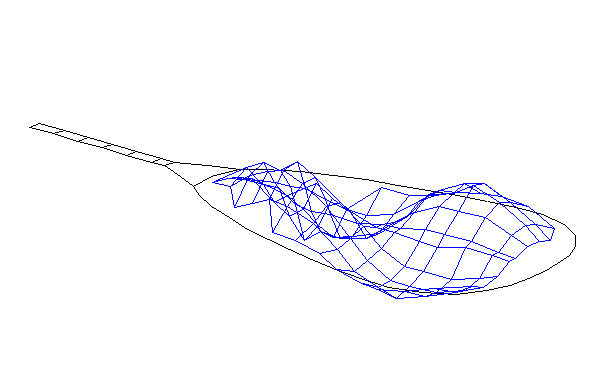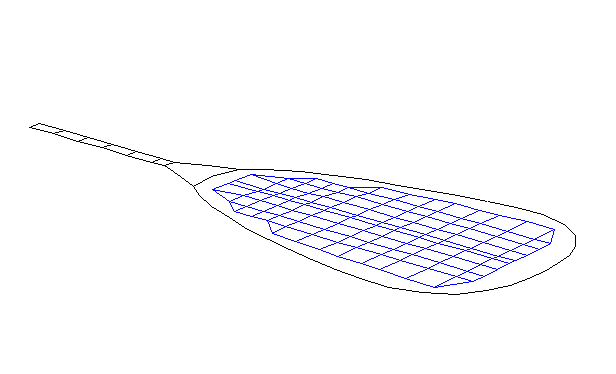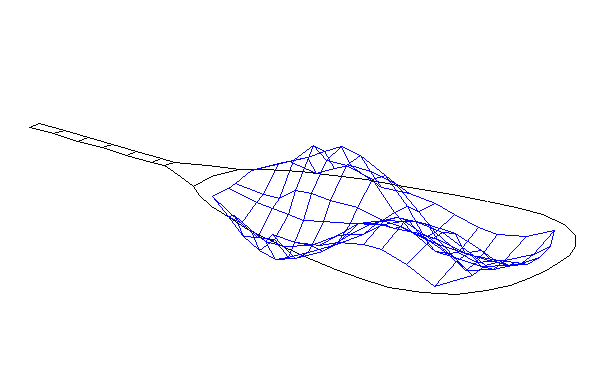
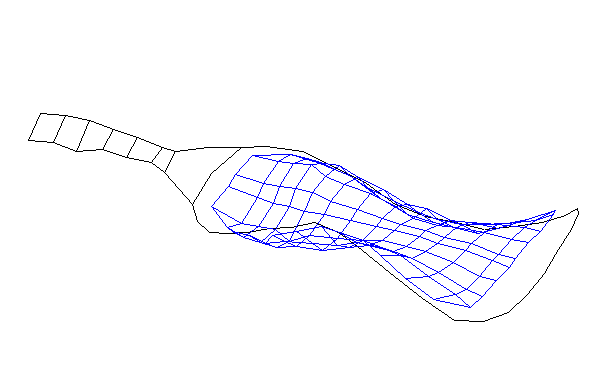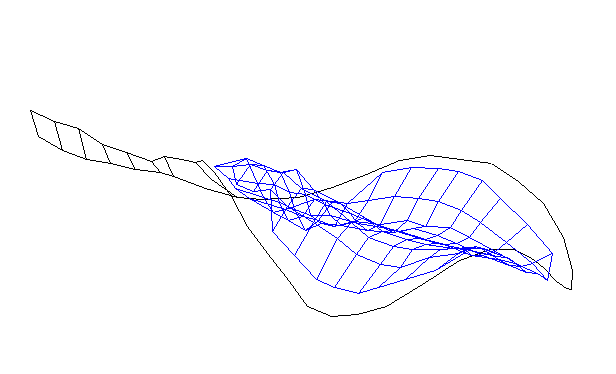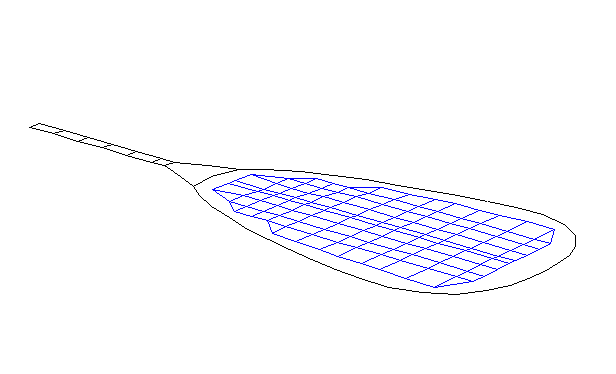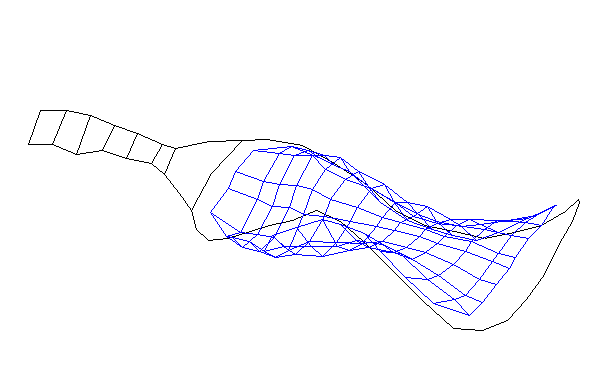
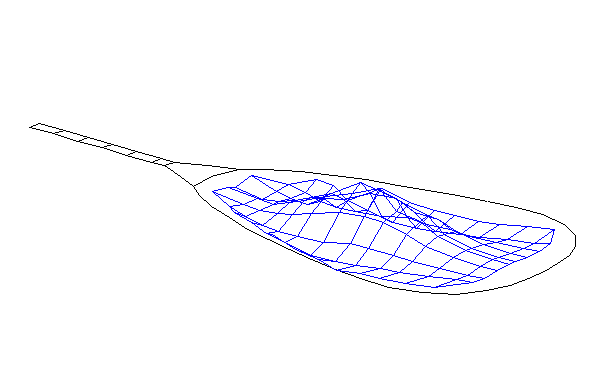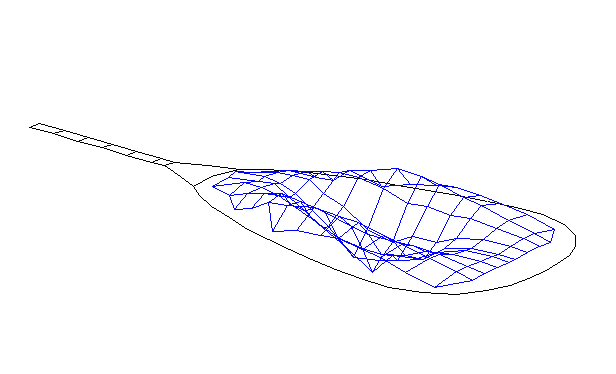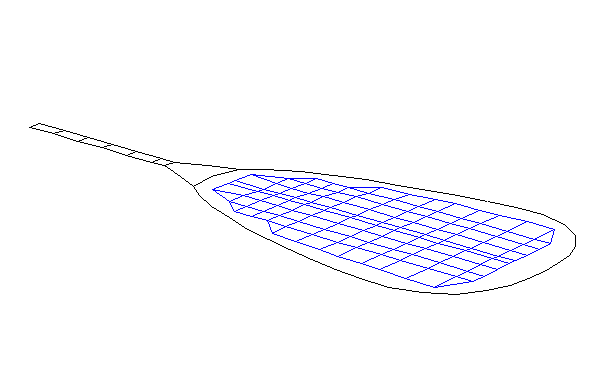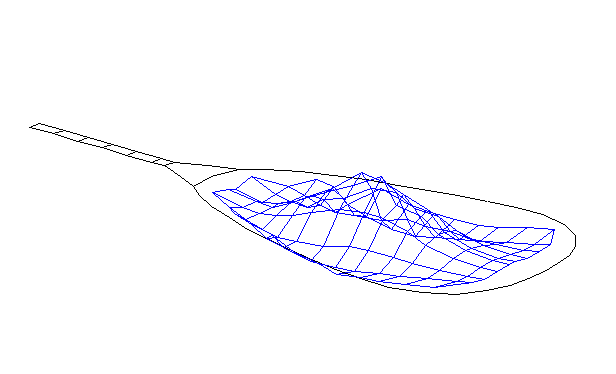
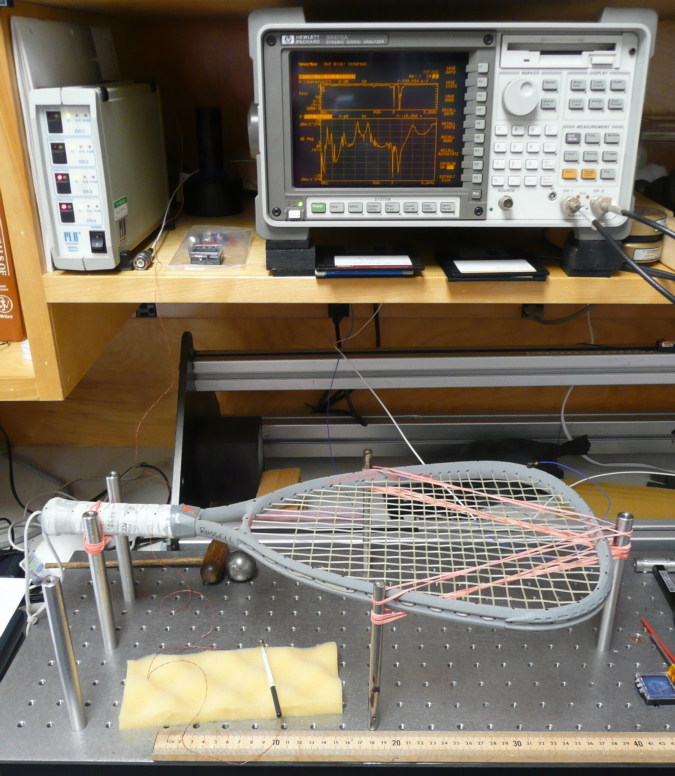
The Experiment and the Racquet In the acoustics laboratory at Kettering University, we use a technique known as Experimental Modal Analysis (EMA) to extract vibrational mode shapes, resonance frequencies and damping rates for a structure. The experiment involves tapping a structure with a special hammer that has a force transducer in the tip to measure the impact force as a function of time. The resulting vibration response of the structure to this impact is measured with a small accelerometer attached to the structure. A two-channel frequency analyzer is used to capture the force and acceleration time signals and transform them to frequency spectra. The ratio of acceleration output to force input is called a Frequency Response Function, and is a complex quantity (in the frequency domain) whose real and imaginary parts provide the frequencies and the damping rates, and the amplitude and phase information necessary to determine the mode shapes. We use a software package, STAR Modal, to post-process the data and to produce the mode shape movies shown below. 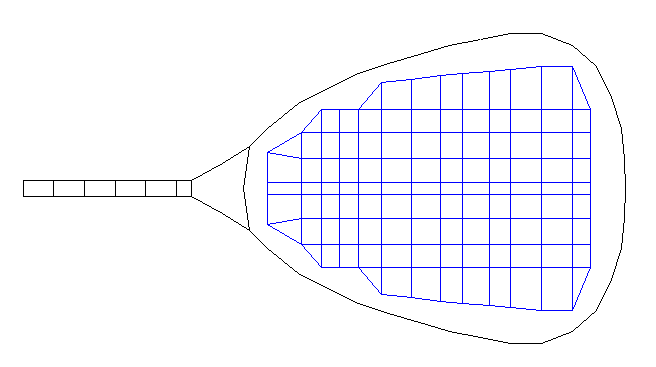The racket in this experiment is a ProKennex Graphite from 1993, one of the early wide body racquetball rackets. I played with this racket while in grad school. The head of the racket (shown at far right) is kind of triangular in shape. The racket measures 20-inches in length. Though shorter than most current rackets, it was one of the first large head rackets introduced in the 1990's. The rackets was marked with impact locations at 1-inch intervals around the frame, and across the strings. A miniature impact hammer (PCB Model 086E80) was used to tap the racket at each impact location, and a small 0.5-gram accelerometer attached to the frame measured the vibration response.

Structural Modes (Frame Modes)		Membrane Modes (String Modes)
There are a number vibrational modes in which the entire racket vibrates as one solid object - strings and frame together. These modes are generally grouped into two types, flexural bending modes and torsional modes.		There are also a large number of vibrational modes in which the frame is pretty much immobile and the strings vibrate in a manner similar to a circular membrane.
	First Bending Mode - 103 Hz.		(1,0) String Membrane Mode - 369 Hz.
	Second Bending Mode - 285 Hz.		(1,1) String Membrane Mode - 574 Hz.
	First Torsional Mode - 174 Hz.		(1,2) String Membrane Mode - 785 Hz.
	Second Torsional Mode - 629 Hz.		(2,0) String Membrane Mode - 838 Hz.

Back to the Acoustics and Vibration Animations Page