
|
Physics and Acoustics of Baseball & Softball Bats
Daniel A. Russell, Ph.D. Graduate Program in Acoustics The Pennsylvania State University The contents of this page are ©2003-2011 Daniel A. Russell | 
|

|
Physics and Acoustics of Baseball & Softball Bats
Daniel A. Russell, Ph.D. Graduate Program in Acoustics The Pennsylvania State University The contents of this page are ©2003-2011 Daniel A. Russell | 
|
This article is a slightly revised lay version of a paper I presented at the 148th Meeting of the Acoustical Society of America, San Diego, CA, November 18, 2004.
| Will the Real Sweet Spot Please Stand Up? | |
|
Trying to locate the exact sweetspot on a baseball or softball bat is not as simple a task as it might seem, because there are a multitude of definitions of the sweetspot:
| |
| Before we take a look at some of these definitions of the sweet spot, I should mention that bat manufacturers often advertise that aluminum bats have a wider sweet spot than do wood bats, or that a particular model of bat is more forgiving for hits away from the sweet spot than competetor models. This is difficult to verify, because of the variety of sweet spot definitions. One batting cage study[1] found that aluminum and wood baseball bats have similarly sized sweet spots, as defined by the range of locations on the barrel which produced the highest batted-ball speeds. While the widths of the sweet spot regions were the same, the aluminum bats hit balls faster from the sweet spot, and also hit balls faster for impacts away from the sweet spot. |
 Figure 1. Width of the sweet spot for metal and wood bats is the approximately the same. |
| The Center-of-Percussion | |
|
The center-of-percussion (COP) is a location that has long been identified as the sweetspot of a baseball bat,[1] and is often identified as such in physics textbooks.[3-5] The COP is determined[6] by pivoting the bat about a point on the handle 6-inches from the knob and measuring the time (the period of oscillation) required for the bat to swing back and forth through one cycle. The COP is strongly influenced by the moment-of-inertia and the distance between the pivot point and the center-of-mass. The center-of-percussion is also known as the center-of-oscillation, because the period of the oscillation of the bat about the pivot point is the same as that of a simple pendulum with the same total mass as the bat, and with a length equal to the COP.
What makes the COP special is that an impact at the COP will result in zero net force at the pivot point. Impacts closer to the handle will result in a translational force at the pivot. Impacts closer to the barrel end will result attempt make the bat rotate about its center-of-mass, causing a force in the opposite direction at the pivot point. However, for impacts at the COP these two opposite forces are balanced, resulting in a zero net force. The COP would seem to be a likely candidate for the sweetspot since an impact at that location would result in zero force at the batter's hands (the top hand is right about 6-inches from the knob). However, the COP is not a fixed point on the bat, but depends on the location of the pivot point. All current methods of testing baseball and softball bat performance use the 6-inch point as the pivot point, and thus as the reference for locating the COP. Three recent studies[7-9] have conclusively shown that at the moment of impact between bat and ball, the actual pivot point is about 2.5 inches beyond the end of the handle, and about 1.5 inches off the axis of the bat. As the pivot point moves beyond the end of the handle the COP moves towards the handle. This means that the pivot point typically used to identify the COP on a bat and the actual pivot point during the collision are not the same - and the COP referenced to the 6-inch point on the handle has little relevance to the collision between bat and ball. | 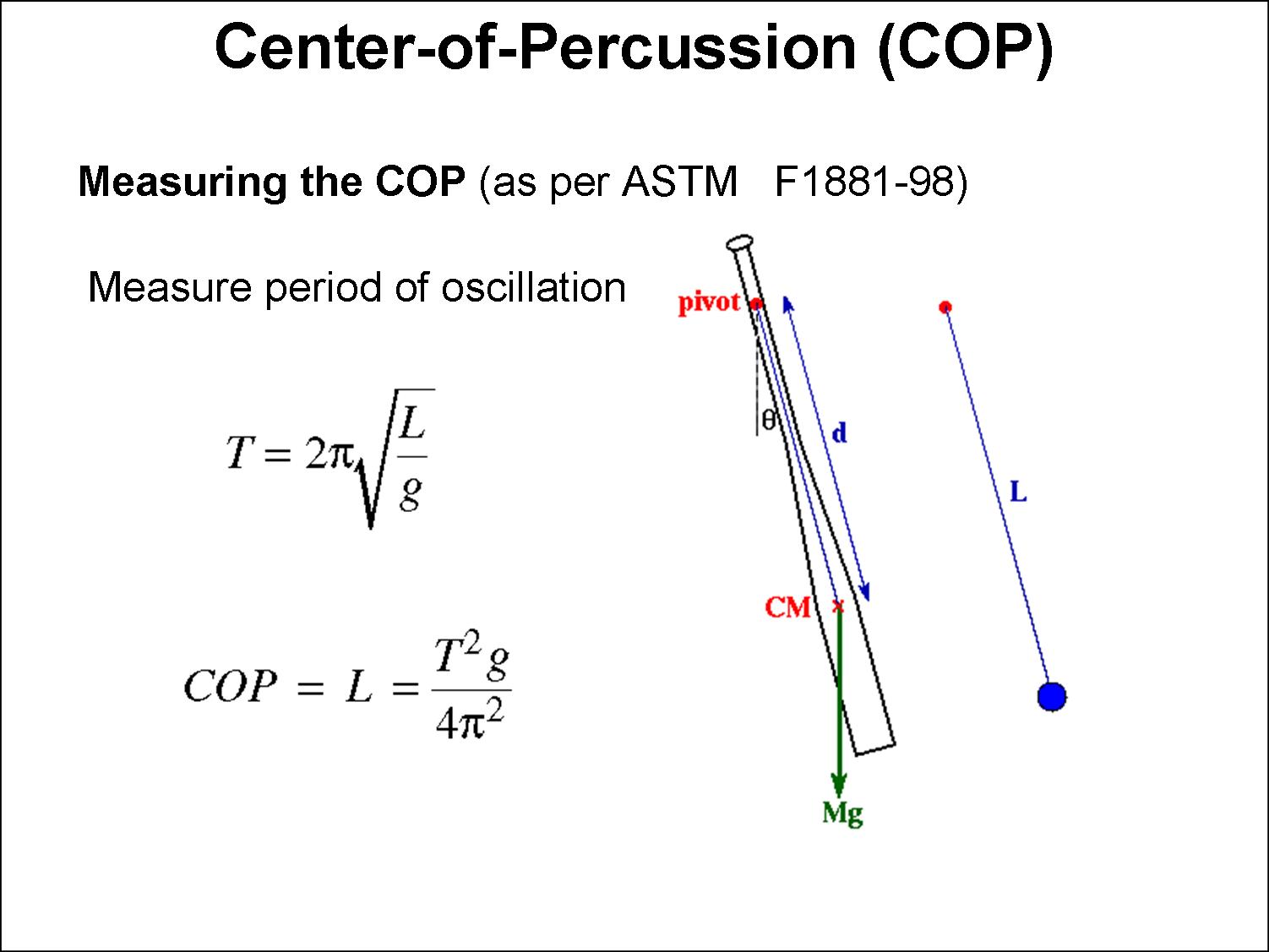 Fig. 2 COP is calculated from measurement of period of oscillation about the pivot point.
|
| Nodes of the First Two Bending Modeshapes | |
|
Another location which has long been associated with the sweetspot is the barrel end node of the first bending mode of vibtation,[10-11] though this location has primarily been associated with the feel in the batter's hands, and less so with the performance sweetspot. The animations in Fig. 4 show the first two bending modes of a high performance slow-pitch softball bat. The first bending shape (120 Hz) has a node approximately 7.5 inches from the end of the barrel. A node represents a point on the bat that does not move while the rest of the bat is vibrating; an impact at a node will not excite this particular mode of vibration. The second bending shape has a node approximately 4 inches from the barrel end of the bat. Thus, one could define a "sweet zone" region between 4-7 inches from the end of the bat; an impact within this region will minimally excite the first two bending modes of vibration. An impact within this region would feel "sweet" because it would not cause the bat to vibrate nearly as much as an impact at the barrel end or in the taper region.
The mode shapes and frequencies of the first 20 or so bending modes are necessary in order to correctly model the interaction between bat and ball.[12] Often impacts away from the sweet spot are described as less effective because the ball looses energy into the bending modes for impacts away from the nodes. This is a problematic conclusion, however, because the short time span of the collision (less than 0.001 seconds for baseball and about 0.0015s for softball) means that the vibrational patterns are not fully established before the contact between ball and bat is over. The shapes and frequencies of the bat vibration are determined by the shape profile of the bat and boundary conditions at both ends. The ball does not know that the handle exists, or whether it is free, hand-gripped, or clamped, because the vibration impulse returning from the handle end of the bat arrives after the ball has already left the bat. This implies that how hard a batter grips the bat should have no effect on performance, something which has been borne out in a recent experiment.[13] As we will see below, the location of the nodes may influence the perception of feel in the batter's hands, but they cannot influence performance. |
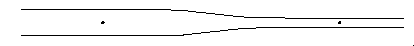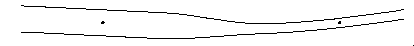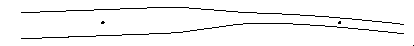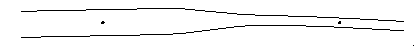 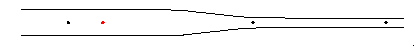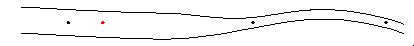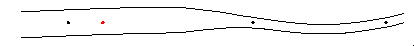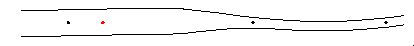 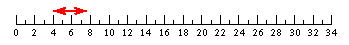 Fig. 4 The first two bending modeshapes for a high performance softball bat. An impact within the region indicated by the red arrow will only minimally excite these vibrational modes.
|
|
A further indication that the node locations are not tied to performance is seen when one compares the locations of the nodes for a free-free bat with a bat which has been clamped in a freely-rotating pivot at the 6-inch point, as shown in Fig. 5. Such a pivoting clamp is used for all current test procedures used to measure performance of baseball and softball bats. The node locations shift, with the node of the first bending shape moving about 1.5 inches away from the barrel end, and the node of the second bending shape moving about half an inch from the barrel end. For the particular slow-pitch softball bat characterized in Fig. 5 the maximum performance was measured at a point 6 inches from the end of the bat, which does not correspond to either of the node locations. | |
| Location of Maximum Performance | |
|
So, we have seen that a definition of the sweet spot as the location of maximum performance does not coincide with either the center-of-percussion or the nodes of the bending shapes. Where is the spot on a bat the results in the maximum performance? This is also not a simple question to answer because it depends on how one defines maximum performance. Two often used metrics for comparing performance between bats are the collision effiency, eA (also known as the apparent coefficient-of-restitution) and the Batted-Ball Speed (BBS). The collision efficiency is used to determine the Ball Exit Speed Ratio (BESR) used to cerfify baseball bats for NCAA and highschool use and also to calculate the Bat Performance Factor (BPF) used to certify softball bats for several governing bodies. The Batted-Ball Speed is the current standard used to certify bats for the Amateur Softball Association. The collision efficiency is essentially determined by the relative elastic properties of the bat and ball, and is measured by firing a ball from a cannon towards a bat and measuring the incoming and outgoing speeds of the ball. Then, The Batted-Ball Speed is calculated from the collision efficiency, but also depends on the moment-of-inertia of the bat, and the impact location where the ball strikes the bat. | |
|
As an interesting historical sidelight, for several years the BPF measurement of a bat's performance was measured at the COP of the bat referenced to the 6-inch point on the handle. Then it was discovered that one could manipulate the moment-of-inertia of the bat so that a given bat would pass the certification test in the lab when tested at the COP. However, that same bat would perform higher than the maximum allowed performance when used in the field. Now all testing methodologies require that the impact location is scanned along the barrel in order to find the position where the performance is maximum before making measurements for certification.
The data in Fig. 6 compares BBS and eA values for three bats at several locations. Notice that the two metrics are not maximum at the same location. For the very high performance composite bat the two maximum locations for the two metrics are separated by 2 inches. Notice also that the maximum for either metric does not occur at the same location for all three bats. There is often a 1.5 to 2 inch variation in the location of the maximum performance between different types of bats. I should also mention that for all three bats, neither of the performance maxima line up with the COP or the nodes of the bending shapes. This data suggests that he location on the bat where performance is a maximum cannot be calculated as a single location for all bats. It must be measured for each individual bat. | 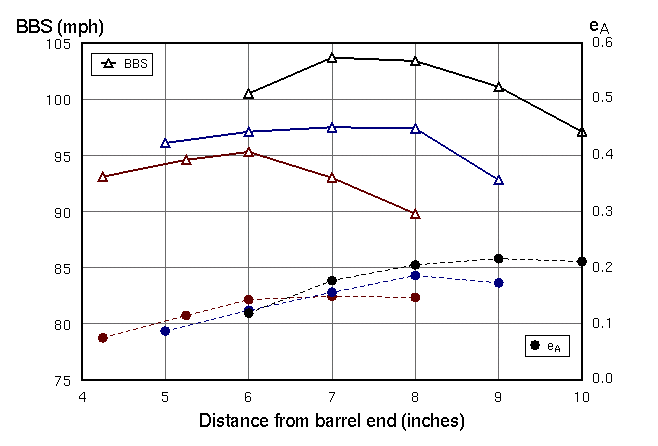 Fig. 6 Comparison of Batted-Ball Speed and collision efficiency (eA) as a function of position for three slow-pitch softball bats: (red) a good single-walled bat, (blue)a high performance double-walled bat, and (black) a very high performance composite bat. |
| Location of Minimum Hand Sensation | |
|
This definition is probably what one thinks of first when referring to the sweet spot.[10] Where on the bat barrel should one hit the ball so that the hands don't feel any vibration or sting? There is some disagrement over whether the node of the first bending shape alone determines the sweet spot,[14,9] or whether it is a combination of the nodes of the first two bending shapes.[11] There are those who hold that it is the region between the node of the first bending shape and the COP that feels the best.[15-16] However, we have seen that since the COP depends on the location of the pivot point it is not a contributor to a working defintion of the sweet spot.
The question would seem to be, then, which is more imporant - the first bending shape, the second bending shape, or both? Figure 7 relates the positions of the hands on the handle to the bending shapes. The top hand is located at a node for the first bending shape and an antinode for the second bending shape, while the bottom hand is at near an antinode for the first bending shape and a node for the second bending shape. Players tend to distinguish between two types of discomfort in the hands for hits away from the sweet spot. Hits at the barrel end of the bat tend to produce a buzz in the lower hand, while impacts near the taper region tend to result in a sharp pain in the fleshy area between thumb and forefinger of the top hand. Comparing the locations of the nodes and the hand positions suggests that the lower hand buzz is caused by the lower frequency first bending shape, while the sting in the top hand is due to the second bending shape. |  Fig. 7 First two bending modes shapes and the locations of the hands. |
|
There are several aluminum bat models with vibration reduction mechanisms which attempt to reduce the vibration which causes hand discomfort. Most of these todate have focused on reducing the vibration of the first bending mode, because it has the greatest amplitude of vibration. If you grip a normal aluminum bat lightly in one hand, at the top hand location on the handle, and tap the barrel end of the bat on the floor you will feel a buzz in your whole due to the first bending mode. Your hand is straddling the node for the first bending mode and and as the bat vibrates your hand feels the bat "rocking" back and forth about the node location. Doing the same with a bat that has a vibration reduction system results in significantly less buzzing sensation. Gripping the bat with both hands kills the vibration so the comparison is not effective. The surprising thing is that bats which effectively damp out the vibration of the first bending mode still cause sting in the top hand for hits at the taper.
New experimental evidence in our laboratory suggests that players overwhelmingly prefer bats with higher vibration reduction for the second bending mode, claiming that a such bats stings much less. | |
| The Hoop Mode and the Sweet Spot | |
In addition to the bending vibration shapes, hollow aluminum and composite bats have an additional set of vibrational shapes which wood bats do not. The cylindrical barrel of a hollow bat exhibits a number of "hoop" modes in which the cross section of the barrel alternataly compresses and expands. The first of these hoop modes is responsible for both the "ping" sound an aluminum bat makes as well as the "trampoline effect" which allows metal and composite bats to significantly outperform wood bats. In fact, the frequency of the first hoop mode correlates very closely with the performance of a hollow bat.[17]
 The displacement of the first hoop mode is not uniform over the entire length of the barrel. Instead, as shown in Fig. 8, the region of maximum displacement occurs roughly 4-10 inches from the end of the bat. This region encompasses the locations of the nodes of the first two bending modes. At the time of this writing, an attempt is being made to correlate the profile of the first hoop mode shape and the location where the performance (either BBS or eA) is a maximum. The displacement of the first hoop mode is not uniform over the entire length of the barrel. Instead, as shown in Fig. 8, the region of maximum displacement occurs roughly 4-10 inches from the end of the bat. This region encompasses the locations of the nodes of the first two bending modes. At the time of this writing, an attempt is being made to correlate the profile of the first hoop mode shape and the location where the performance (either BBS or eA) is a maximum.
At first glane it would appear that because the hoop modes do not involve any vibration in the handle of the bat, they should not influence the sensation of feel in the hands. However, anecdotal evidence suggests that higher performance softball bats sting less. This could very well be due to the lower hoop frequency responsible for the higher performance. The lower the hoop frequency, the softer the spring constant of the bat, and the contact time with the ball slightly increases. While the impulse (change in momentum) delivered to the ball does not decrease, the slightly longer contact time results in a lower peak force delivered to the bat, which in turn means a smaller vibration amplitude in the rest of the bat. |  Fig. 8 Comparing the mode shape of the first two bending modes with the first hoop mode.
|
In some sense we have come full circle to the problem stated at the beginning of this paper, that there is no single definition of the sweet spot for a hollow baseball or softball bat. There are locations on the barrel which result in maximum performance and there are locations which result in minimal discomfort in the hands. These locations are not the same for a given bat, and there is considerable variation in locations between bats. Hopefully this summary will enhance the understanding of what the sweet spot is and what it is not, as well as encouraging further research into the quest for the "perfect bat."
Back to Physics and Acoustics of Baseball & Softball Bats