Today is
This article was originally posted on January 3, 2003.
The contents of this page were last modified on May 28, 2012
Does it matter how tightly you Grip the Bat?
Should you grip the bat handle as hard as you can, or loosely as you swing? Does gripping the bat harder make the ball come off the bat faster? What effect (if any) do the hands have on the bat-ball collision? Does a laboratory test which clamps a bat in a pivot at the handle produce results which are similar to what happens when a player swings a bat at a pitched ball? These are important questions, both from a player/coach perspective, but also from a testing/standards issue. All of the current test procedures used to certify which bats are legal for play in various leagues use a laboratory test in which the bat is clamped at the handle in a pivot which is free to rotate. It is reasonable to question whether or not this adequately simulates a player swinging a bat. What follows below is a chronological summary explanation and analysis of several published research papers I have found which address the subject.
Evidence #1: ball rebound speed from free and clamped bats
One of the earliest attempts to scientifically answer these questions regarding hand grip appears to be an experiment carried out in the late 1980's by a group of researchers in the Department of Health and Sports Science at Wake Forest University.[1] They used a pitching machine to fire balls towards the bat, from about 5 feet away, with a pitched ball speed of 60 mph, and simply measured the rebound speed of the ball after hitting the bat. They looked at two extremes of simulated grip conditions: free and clamped. In the free case the handle of the bat was suspended by a length of fishing line attached 7 inches from the handle while the barrel end of the bat rested on a knife edge. The 7-inch point was chosen based on an experiment by Noble and Eck[2] who found that the axis of rotation of the bat handle during impact was at about the location of the index finger of the top hitting hand.[3] For the clamped case the handle of the bat was firmly secured in a vise so that the entire 7-inches of the handle, starting at the knob, was enclosed in the vise grip and not allowed to move. They measured the rebounding ball speed for both an aluminum and a wood bat at three locations, the center-of-mass, the center-of-percussion, and the barrel end of each bat. They reported that (i) impacts at the Center-of-Percussion resulted in the greatest post-impact ball speed, (ii) balls rebounded faster from a tight grip (clamped bat) than from a non-tight frip (free bat), and (iii) wood bats produce higher post-impact ball speeds than do aluminum bats. The fact that they claim wood bats outperform aluminum bats, completely the opposite result of several other carefully conducted tests and experiments,[4] is alone enough to suggest that there are some problems with this early experiment to asses the effect of hand grip. In addition, recent studies have shown conclusisvely that the center-of-percussion has absolutely no bearing on bat performance,[5] overturning their first conclusion. So, one might also suspect that their second conclusion regarding the effect of hand-grip might also be questionable.
I have some problems with the way the data was presented in this paper, primarily in the way they lumped the data from both wood and aluminum bats and the three impact locations together and tried to use statistics to reveal trends without separating bat types or impact locations individually. However, the more significant problem to me is the difference in the behavior of the bats in the two end conditions they tested. The free bat was suspended from the handle using fishing line while the barrel rested on a knife edge. After impact from the ball the bat is completely free to translate (swing away) and rotate about its center-of-mass (or a compromise between the CM and the pivot point). This "recoil" of the bat is significant because conservation of momentum says that any post-impact velocity of the bat changes the post-impact velocity of the ball. For the clamped bat, the entire handle of the bat was rigidly clamped in a vise so that the bat could flex or bend, but could not rotate or recoil after impact. It is my belief that this is an unrealistic boundary condition, and that all three of the results of this paper are in error. So, while this experiment is part of the history of bat research, I don't believe that it lends any useful answers to the question of how gripping the bat affects performance.
Evidence #2: frequencies of free, clamped, and hand-held bats
A more reliable experiment was carried out in 1990 by tennis science expert, Howard Brody[6] included data for hand-held baseball bats. He compared the vibrational behavior of wood and aluminum bats which were free at both ends, clamped at the handle end, and hand-held. He mounted a strain gauge on a wood bat and struck it with a baseball and recorded the resulting vibration response of the strain gauge on an oscilloscope. He found that the clamped bat exhibited what is called a "diving board" mode at a very low frequency of 18 Hz, as well as a higher frequency, second mode at 209 Hz. The free bat, however, did not exhibit this diving board mode at all, but instead its lowest vibrational mode was similar to a free-free beam, and had a frequency of 163 Hz. He found different frequencies, but the same trends for an aluminum bat. The animations below illustrate what the first two diving board and the first free-free mode shapes look like. The relative speeds with which the aminations is similar to the ratio of frequencies Brody measured.
1st diving board mode for a clamped beam
|

2nd diving board mode for a clamped beam
|
1st bending mode for a free-free beam
|
When Brody measured the vibration of hand-held bats (both wood and aluminum) he found absolutely no evidence of the low frequency diving board vibration. For both tight and loose hand grips he found only a higher frequency vibration, very close to frequency of the the free-free bending mode. However, this vibration was severely damped by the hands and died away very quickly, with a tight grip damping the vibration more quickly than a loose grip. His conclusion was that "a freely suspended bat corresponds to both the loosely held and very tightly gripped bat, and a bat firmly clamped in a vise does not behave the way a hand-held bat does." One implication of this conclusion is that a player could essentially throw the bat at the ball and it would have the same effect as gripping the bat tightly in an attempt to get more power out of the collision with the ball.[7]
Evidence #3: superballs and aluminum beams
A fascinating simple experiment was carried out about five years ago by another tennis science expert: Rod Cross.[8] Cross fired superballs against aluminum beams of various lengths and measured the apparent coefficient-of-restitution (ratio of outgoing to incoming ball speed). Quoting from Cross' paper: "The results in Fig. 6 show very clearly that, for a sufficiently long beam, (a) the impact of a ball near one end of a beam is not affected by the length of the beam or the method of support at the other end and (b) the ACOR for an impact anywhere along the central section of a beam is independent of the impact location and is not affected by the length of the beam or the method of support." The figure below shows two of the graphs from Fig. 6 in Cross' paper. The dots in the figure are measured data points and the smooth curve is a theoretical prediction. The figure shows very nice agreement between theory and experiment. The figure also shows that for impact locations from the center of the beam to the free end (55cm to 110cm) there is no difference in the two plots. This suggests that impacts at the barrel end of a baseball bat are completely unaffected by how the handle of the bat is gripped. The plots also show that over the entire middle section of the beam (20cm to 80cm) the coefficient-of-restitution is the same; the ends of the beam only begin to affect the impact once you get closer to the end than about 20% of the entire length of the beam. This suggests that for impacts along the entire hitting area of a baseball bat, the handle should have absolutely no affect. Only for impacts right on the handle would grip matter.
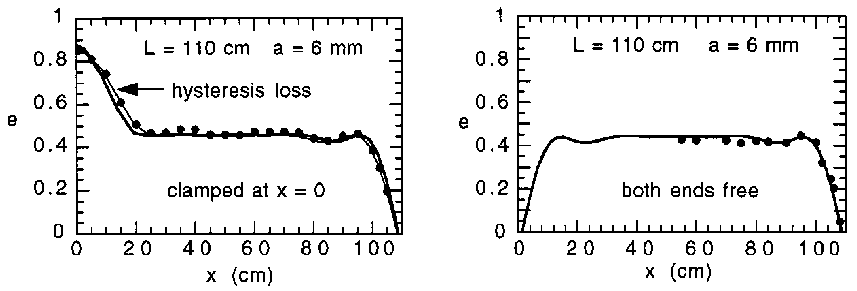
Exerpt from Fig. 6 of Cross' paper[8] comparing apparent coefficient-of-restitution for impacts near one end of a free-free beam with impacts near the free end of a clamped-free beam. The measured results (on the right half of each graph) are the same, even though the boundary conditions at the other end of the beam are very different.
|
The explanation behind this result is that it takes time for vibrational energy to travel from the impact point, along the beam (or bat) to the end, reflect from the end, and return to the impact point. If the impact occurs close to the end, then the travel time to the end of the beam (or bat) is short enough that the impulse returns before the collision between ball and bat is over, and the collision is affected by the return of this vibrational energy. However, for impacts far enough away from the end, the impulse cannot not reach the end, reflect, and return before the collision is over. The ball breaks contact with the beam (or bat) before it even knows that the end exists.
Evidence #4: Computational model of bat-ball dynamics
|
Alan Nathan has developed an elegant computational model[9] of the bat-ball collision that supports the idea that the collision between bat and ball is over before any information about the handle end of the bat is available to the ball. Nathan differentiates between the collision time, during which 99% of the impulse is delivered to the ball to change its direction and speed, and the contact time which is the total time the bat and ball are in contact with each other. Because of hysteresis effects in the ball it is possible for the ball to be in contact with the bat even though no forces are being exerted on the ball. Based on experimental data, Nathan calculates uses a incoming ball speed of 67 m/s to obtain an approximate contact time of 1.1 ms with a shorter collision time of 0.6 ms. His computational model allows him to analyze the vibrational behavior of the bat during and after the collision, and he was able to show that under realistic playing conditions the collision time between bat and ball is long over before any information from the handle end of the bat is available to alter the collision. The figure at right shows the velocity response (as a function of time) of the handle of the bat after an impact on the barrel. The arrow identifies the time at which 99% of the impulse has been delivered to the ball. The plot shows that the handle is only just beginning to respond to the impact by the time the collision is over. I have measured similar response plots in my laboratory and the propagation time - the time it takes for a vibrational impulse to travel from the impact location to the handle and back again - is longer than the impact duration. This means that there is not possible for the way in which the handle is gripped to influence the final ball speed since the ball has already left the bat before it even knows the handle exists. As far as the ball is concerned, it has just impacted an infinitely long bat with no ends (with the same inertial properties as the real bat, of course). Only for impacts very close to the handle can the manner of gripping possibly affect the resulting batted-ball speed.
|

Figure 15 from Alan Nathan's paper[9] showing the velocity response as a function of time for a point on the handle of the bat 13cm from the knob due to an impact in the barrel (68cm from the knob). The arrow indicates the time at which the collision is over - the change in momentum of the ball is 99% complete. Notice that the handle has just barely begun to move by the time the collision between bat and ball is complete.
|
Evidence #5: batted-ball speed for pivoted, swung (hand-held) and free bats
So far we've looked at a baseball bat experiment with very questionable results, an experiment which compared the vibrational frequencies of bats in different grip conditions, a simple experiment bouncing superballs off aluminum beams, and a computational model of the bat-ball collision. The last three studies agree in their predictions that grip conditions should have no affect on bat performance. However, is would be really nice if there was an experimental study players and realistic grip conditions. Fortunately such a study exists! In September 2004 I attended the 5th International Conference on Engineering of Sports and saw a very nice paper presented by Keith Koenig[10] and his colleagues from the University of Mississippi. Koenig's laboratory is responsible for some of the early research which led to the development of the current ASTM test methods for comparing and measuring bat performance. They conducted the most comprehensive study I have seen so far regarding the effect of hand grip on baseball performance. They investigated three grip conditions. The first condition was free-free, with the bat simply balancing on two small posts about 9.5 inches from each end of the bat. Upon impact the bat was free to go flying away. The second grip condition was modeled after the clamped-pivot used for ASTM testing of bats. The bat was clamped in a fixture about two inches wide and centered at a location on the handle; two locations were chosen: 4.75" and 5.75" from the knob (ASTM standards use a location 6" from the knob). The pivot was completely free to rotate about a fixed axis, allowing the bat to recoil and pivot about this axis, but not translate. The third condition was a hand-held grip in which four players (three experienced college and one semi-professional) swung the bat at a ball on a tee. In the first two cases (free and clamped/pivot) they fired balls from an air compression cannon towards a stationary bat, with incoming ball speeds varying between 50 and 110 mph. For the hand-held/swing-hit case they measured the bat swing speed at the impact location just prior to impact with a stationary ball. In all three cases care was taken to ensure that all impacts occured at the same location on the bat barrel, approximately 4-inches from the end of the barrel. This ensured that any differences in "batted-ball speed" would be due only to differences in grip conditions, not due to variations in impact locations.
The data in the figure below shows the combined results of all three tests (two pivots, free-free, and swing-hit). The fact that all of the data points appear to fall on a straight line indicates that grip conditions have absolutely no affect on batted-ball speed. If grip did affect performance, then we would expect to see three or four straight lines, one for each data set. Koenig's results, however, show that there is no noticeable difference in the performance of a bat which is clamped in pivot, free-free, or gripped with the hands and swung by a player.

Koenig's measured batted-ball speed as a function of pitched-ball speed (or bat swing speed) for a variety of grip conditions.
|
Evidence #6: Video of "No-Hands" Homerun
On May 27, 2012 Todd Frazier hit a solo home run off Jamie Moyer in a game during which the Rockies and Reds combined for a ballpark record of 9 home runs. What was unique about Frazier's home run was the fact that he lost grip of the bat just before the bat and ball made contact. The bat was slipping out of his hands as he swung, and was essentially free as it impacted the ball. The bat flew out toward the pitcher's mound, about 60 feet from home plate while the ball goes over the fence for a home run. In the slow-motion segment of the video (from Fox Sports) you can clearly see that his right hand has lost contact with the bat before impact with the ball, and the bat is slipping out of his left hand at the moment of impact. This doesn't happen very often, but confirms the physics described above that a player could "throw" the bat the ball - or in this case, release it in the instant before impact - and the result of the bat-ball collision would be the same.

Conclusion: Final batted-ball speed does NOT matter how you grip the bat
So, what have we learned? Well, we've learned that while gripping a bat in the hands (loosely or tightly) has an affect on the damping rates of the ensuing bat vibration, it does not significantly alter the frequencies with which the bat vibrates - so that a hand-gripped bat behaves more like a free-free bat and not like a bat with a clamped handle. We have also learned that boundary conditions at the end of a bat only affect collisions which occur very close to the end of the bat. Impacts at locations removed from the ends are independent of the manner in which the end is supported. A good part of the reason why this happens is that it takes time for a vibration impulse to travel from the impact location to the handle and back again. Measurements and computer models show that the collision between bat and ball is over before the bat handle has even begin to vibrate and the ball has left the bat before it even knows the handle exists. Finally, experimental evidence comparing the effect of different grip conditions on resulting batted-ball speed conclusively shows that the manner in which the handle is gripped has no affect on the performance of the bat.
References
[1] Andrew S. Weyrich, Stephen P. Messier, Brian S. Ruhmann, and Michael J. Berry, "Effects of bat composition, grip firmness, and impact location on postimpact ball velocity," Medicine and Science in Sports and Exercise, 21(2), 199-205 (1989)
[2] L. Noble and X. Eck, "Emperical determination of the axis of percussion of softball and baseball bats," in Biomechanics IX-B, D.A. Winter, R.W. Norman, R.P. Wells, K.C. Hayes, and E. Patla (Eds.), Champaign, IL (1985) pp. 516-520.
[3] On my web page discussing moment-of-inertia and swing speed I discuss results from three recent carefully conducted field studies which show that the point of rotation during impact is actually about 2.5 inches beyond the knob and about 1.5 inches off the long axis of the bat, and NOT at a point on the handle 7 inches in from the knob.
[4] See my webpage entitled "Why do aluminum bats outperform wood bats"
[5] see my webpage entitled What is the COP (center of percussion) and does it matter?
[6] Howard Brody, "Models of baseball bats," American Journal of Physics, 58(8), 756-758 (1990)
[7] William Brown, "Baseball players throw their bats at the ball," New Scientist, (6 October 1990)
[8] Rod Cross, "Impact of a ball with a bat or racket," Am. J. Phys., 67(8) 692-702 (1999)
[9] Alan M. Nathan, "Dynamics of the baseball-bat collision," Am. J. Phys., 68(11) 979-990 (2000)
[10] K. Koenig, J.S. Dillard, D.K. Nance, and D.B. Shafer, "The effects of support conditions on baseball bat testing," Engineering of Sport 5 Vol. 2, pp. 87-93 (International Sports Engineering Association, 2004). Proceedings of the 5th International Conference on the Engineering of Sport, UC Davis, September 11-15, 2004.
Back to Physics and Acoustics of Baseball & Softball Bats










