The piano hammer as a nonlinear spring
Copyright © 1997 Daniel A. Russell, Science and Mathematics
Department, GMI Engineering & Management Institute
Although the hammers in the very first pianos consisted of wooden heads covered with leather, felt hammers have been standard since about 1830. Modern hammers consist of a wooden molding covered with several layers of compressed wool felt, whose hardness is carefully controlled. In order to produce a good tone, the hardness has a gradient so that the outer surface is softer than the inner layers. This gradient may be adjusted using various techniques in a process called voicing.
The hardness of a piano hammer has a great deal of influence on the resulting piano sound. Hard hammers are better at exciting high frequency modes of a piano string's vibration so that the resulting tone quality may be characterized as being bright, tinny, or harsh. Soft hammers, on the other hand, do not excite high frequencies very well, and the resulting tone is somewhat dull or dark. The static hardness of a piano hammer may be tested by a durometer or hardness tester. In a typical piano, treble hammers are much harder than bass hammers.
Static hardness is not the only factor in determining piano tone. Dynamic hardness also plays an important role. A piano hammer behaves somewhat as a hardening spring; for large impact forces the hammer felt appears harder than it does for low impact forces. In the piano this means that a loud note sounds much brighter (i.e. contains more high frequencies) than a quiet note. It is difficult to test the dynamic hardness of hammers except by listening to them in a finished piano. If the piano tone is not as desired then the hammer hardness must be adjusted by voicing.
Measuring hammer felt nonlinearity from force-compression curves
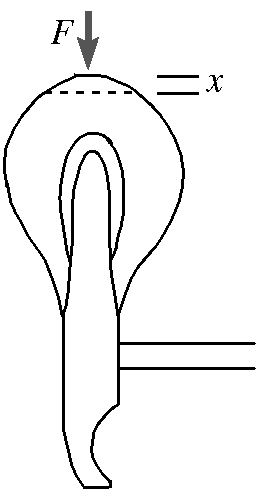 When a force F is applied to the felt of a piano hammer, the felt is compressed. The distance by which the felt is compressed depends on the amount of applied force. If the piano hammer felt behaved like a linear spring then the relationship between applied force F and compression x would be a linear relationship F = k x, where k is a constant depending on the properties of the spring. This linear relationship is known as Hooke's law.
When a force F is applied to the felt of a piano hammer, the felt is compressed. The distance by which the felt is compressed depends on the amount of applied force. If the piano hammer felt behaved like a linear spring then the relationship between applied force F and compression x would be a linear relationship F = k x, where k is a constant depending on the properties of the spring. This linear relationship is known as Hooke's law.
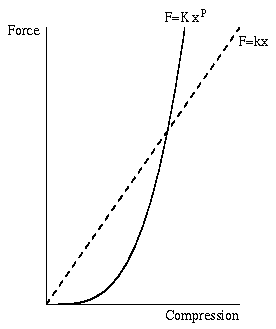 However, when a steadily increasing force is applied to a wool felt pad, the resulting compression does not obey the linear relationship F = k x. Instead, one finds that the felt appears to become stiffer the more it compresses, so that a larger force must be applied to produce the same increase in compression. In this case, the wool felt used for piano hammers acts as a nonlinear hardening spring. Measurements (reported in the literature) of applied force versus felt compression for many different brands of piano hammers show that the nonlinear relationship between applied force and felt compression appears to be of the form F = K x p ,
where K is a generalized stiffness of the hammer, and the exponent p describes how the stiffness changes with applied force. The greater the value of p the greater the range of hammer stiffness for a given range of force. This exponent p is called the effective nonlinearity exponent
However, when a steadily increasing force is applied to a wool felt pad, the resulting compression does not obey the linear relationship F = k x. Instead, one finds that the felt appears to become stiffer the more it compresses, so that a larger force must be applied to produce the same increase in compression. In this case, the wool felt used for piano hammers acts as a nonlinear hardening spring. Measurements (reported in the literature) of applied force versus felt compression for many different brands of piano hammers show that the nonlinear relationship between applied force and felt compression appears to be of the form F = K x p ,
where K is a generalized stiffness of the hammer, and the exponent p describes how the stiffness changes with applied force. The greater the value of p the greater the range of hammer stiffness for a given range of force. This exponent p is called the effective nonlinearity exponent
Static measurements (apply force, measure compression, change force, measure new compression . . .) typically produce values of p ranging from 2.2 to 3.5 for hammers taken from pianos, and 1.5 to 2.8 for unused hammers, with no definite trend from bass to treble.
According to surveys of several performers, the preferred range of values for the nonlinearity exponent is 2< p < 3. If p = 1 the hammer is too linear and loud notes are simply amplified soft notes. If p > 3 there is too much contrast - fortissimos are too harsh and pianissimos are too bland.
Hysteresis
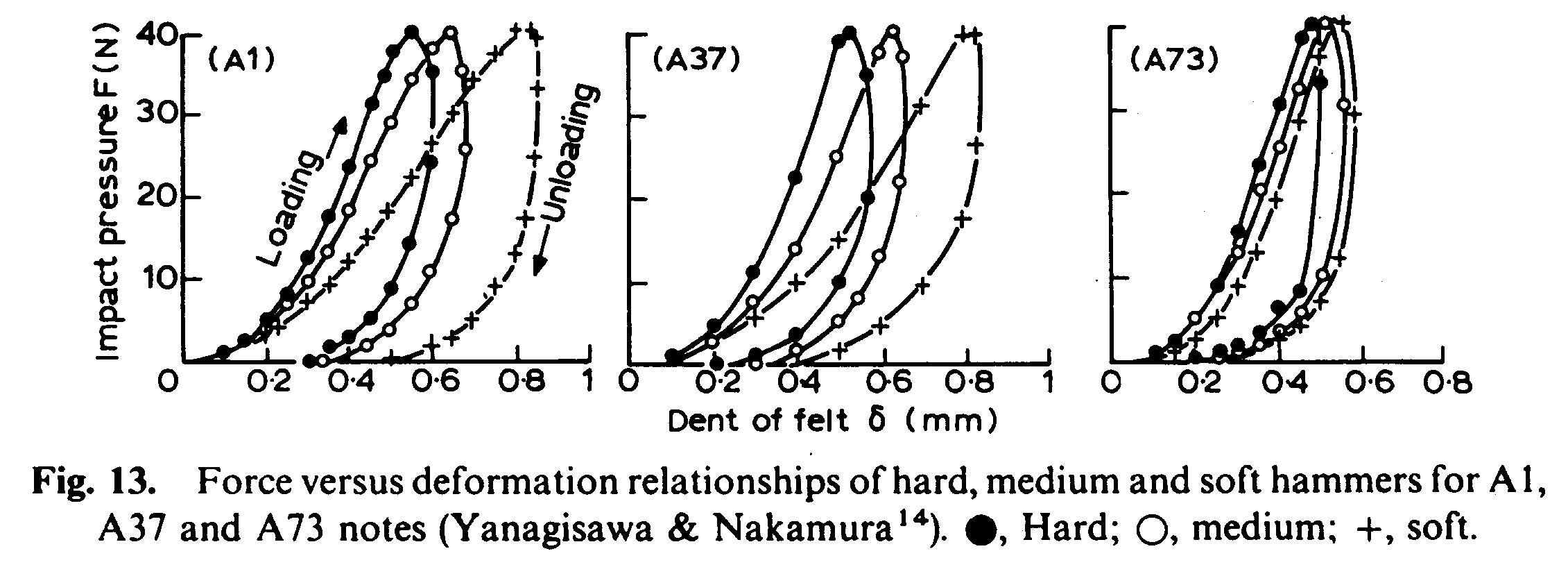
Another interesting feature of piano hammer felt is the presence of hysteresis. Force-compression curves do not follow the same path during compression and relaxation of the felt. Instead a hysteresis loop is formed. This is due largely to slippage of the wool fibers which occurs during slow and large amplitude compression.
Measuring hammer felt nonlinearity from force-time curves
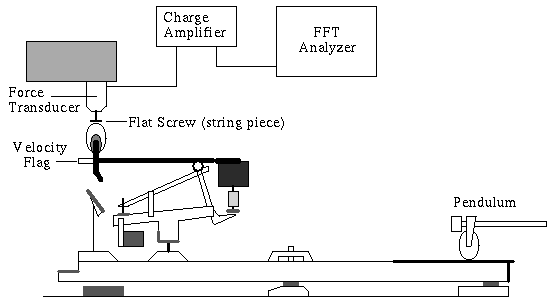 While static measurements of the effective nonlinearity exponent p from force-compression curves are useful, they cannot completely describe what happens during an impact as the hammer strikes the string. In a dynamic measurement, a piano hammer strikes a piece(s) of piano wire attached to the face of a force transducer, securely mounted in a drill press. The force transducer measures the time-history (force versus time) of the hammer impact. Dynamic stiffness can be extracted from this force-time curve as will be shown below. Others (Boutillon, Yanagisawa and Nakamura, Askenfelt and Jansson) also measured the compression dynamically using gap sensors.
While static measurements of the effective nonlinearity exponent p from force-compression curves are useful, they cannot completely describe what happens during an impact as the hammer strikes the string. In a dynamic measurement, a piano hammer strikes a piece(s) of piano wire attached to the face of a force transducer, securely mounted in a drill press. The force transducer measures the time-history (force versus time) of the hammer impact. Dynamic stiffness can be extracted from this force-time curve as will be shown below. Others (Boutillon, Yanagisawa and Nakamura, Askenfelt and Jansson) also measured the compression dynamically using gap sensors.
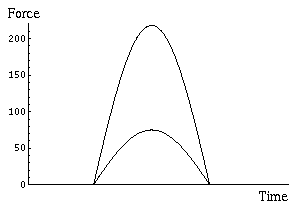
Expectation for a linear hammer
If piano hammers behaved as linear springs, one might expect certain behaviors. First, the shape of the force-time curve should resemble a half-cycle sine curve. Second, the time duration of the impact of the hammer should not depend on the maximum force of the impact. For soft and hard blows the hammer should remain in contact with the force transducer for same amount of time, only the peak force values would be different. Third, the difference between a hard and soft hammer would show up in the contact duration time: soft hammers would remain in contact longer than hard hammers.
| Soft linear hammer |
Hard linear hammer |
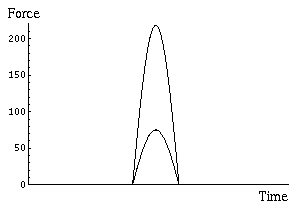
Measured linear hammer
Polyurethane elastomer |
 |
 |
 |
Results for a nonlinear hammer
Measurements of the force-time curve for a real piano hammer show that the pulse shape is not a half-cycle sine curve. The dashed curve in the figure below represents the measured force-time pulse for an A3 hammer with an impact velocity of 4 m/s. The solid curves represent attempts to fit the measured data to (a) half-cycle sine, (b) sine-squared, and (c) the product of a sine-squared and exponential functions. Evidence of hysteresis shows up in that the measured curves are not left-right symmetric. The hammer does not rebound as quickly as it is compressed.
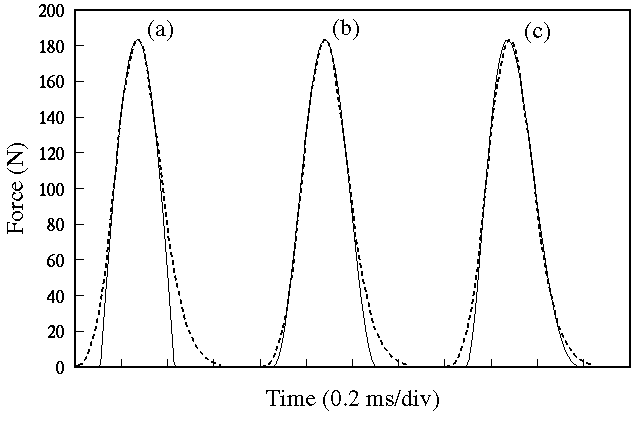
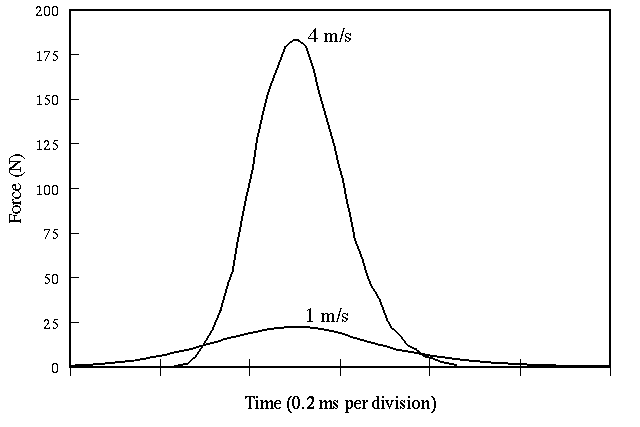
Furthermore, in contrast to a linear hammer, the contact duration of a real hammer decreases for increasing peak force as shown in the figure below (hammer A3 at impact velocities of 1 m/s and 4 m/s). For a real hammer, hard blows have a shorter duration than soft blows.
Extracting the effective nonlinearity exponent p from a force-time curve
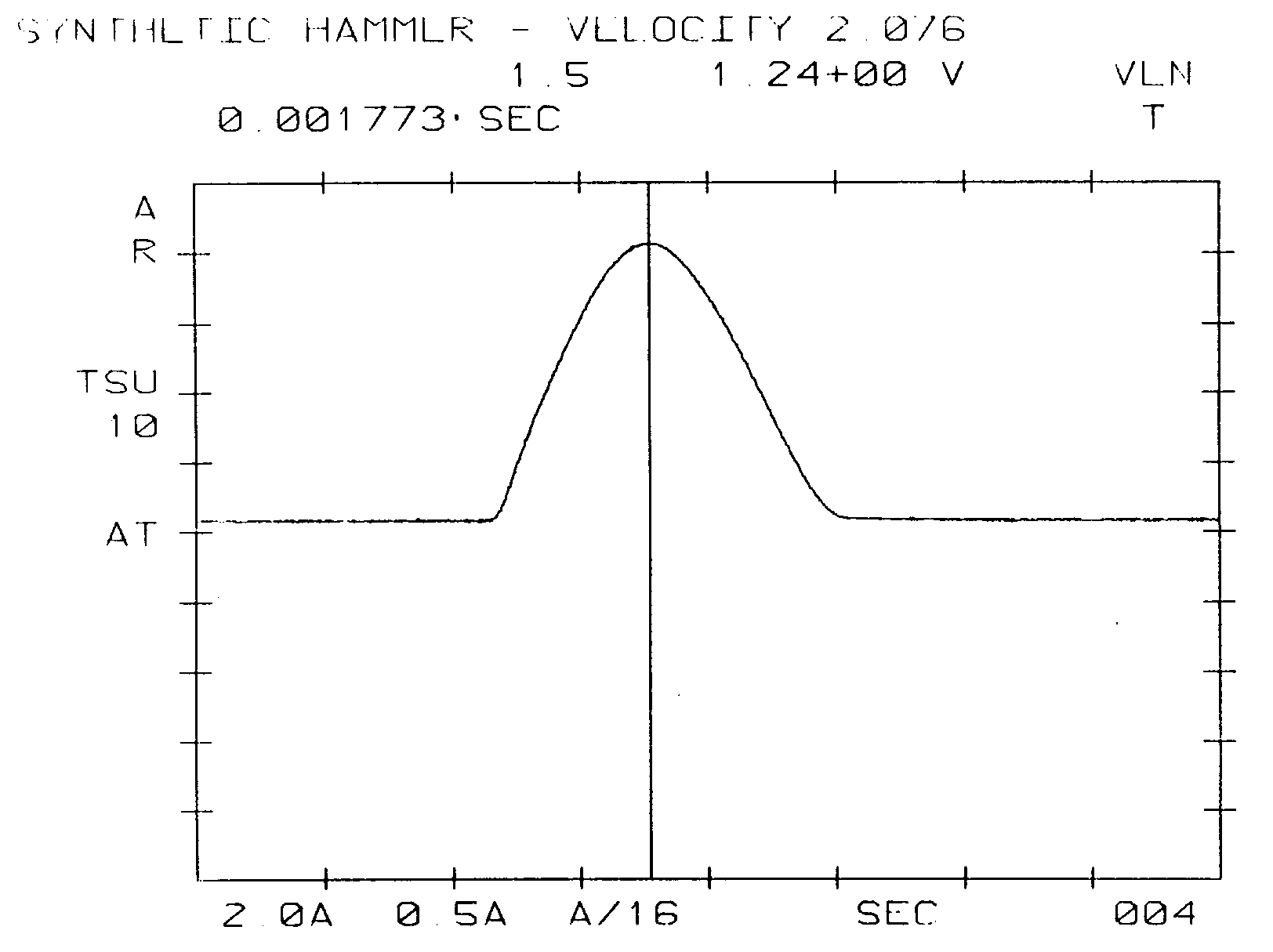
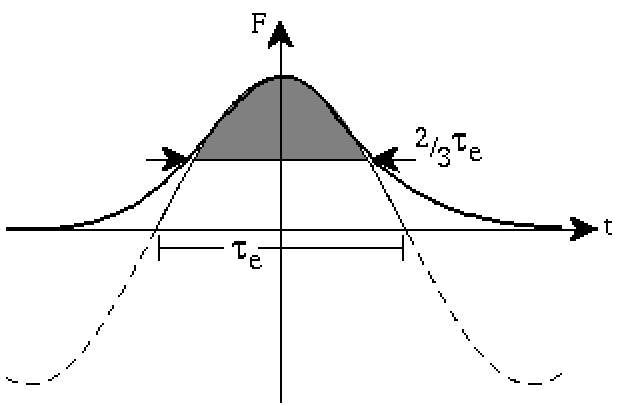 Hall and Askenfelt have developed a method of extracting the effective nonlinearity exponent p from a force-time curve measured from a hammer impact. They attempted to fit the upper portion of the force-time curve with a half-cycle sine curve. An effective stiffness may then be determined from SE = m π2 / τe, where m is the hammer mass, and τe is the effective time duration of the impact. τe is determined by measuring the halfwidth (pulse width at ½ peak) of the force-time curve (see figure at right).
Hall and Askenfelt have developed a method of extracting the effective nonlinearity exponent p from a force-time curve measured from a hammer impact. They attempted to fit the upper portion of the force-time curve with a half-cycle sine curve. An effective stiffness may then be determined from SE = m π2 / τe, where m is the hammer mass, and τe is the effective time duration of the impact. τe is determined by measuring the halfwidth (pulse width at ½ peak) of the force-time curve (see figure at right).
The effective contact duration for a hammer blow on a rigid surface is related to the maximum force by τe ≈ Fmax(1-p)/2p
where the exponent p is the same effective nonlinearity exponent as measured from static force-compression curves.
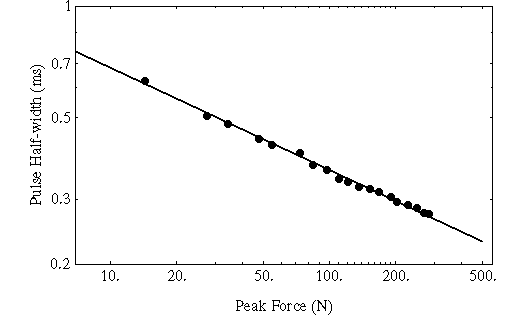
By plotting the pulse half-width versus peak force on a log-log plot (the figure below is for a D2 hammer with p=2.2), one can calculate the exponent p.
The plot below shows exponent values measured for a Steinway voiced hammer action (black) and a set of hammers removed from a Steinway because they were too hard (white).
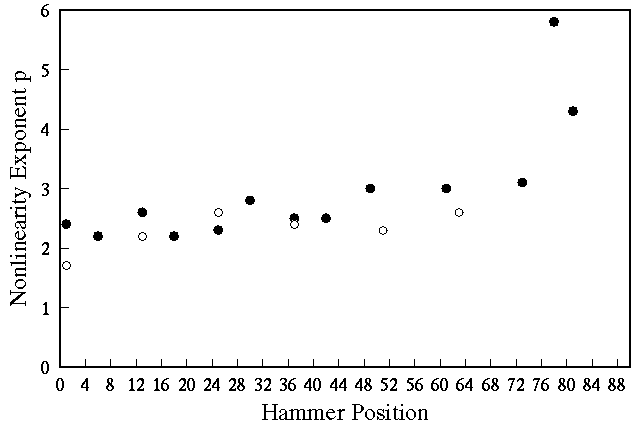
The relatively smooth increase from p≈2 in the bass, to p≈4 in the treble seems to agree with other measurements in the literature. From this figure we might predict that treble hammers will show greater changes in stiffness than bass hammers if both are tested over the same range of velocities. The data also shows that most of the hard hammers have lower exponent values than the voiced hammers, a result that agrees with measurements made by Hall and Askenfelt. This suggests that the hard hammers should show smaller changes in stiffness than the voiced hammers.
Back to the Piano Hammer Page
 However, when a steadily increasing force is applied to a wool felt pad, the resulting compression does not obey the linear relationship F = k x. Instead, one finds that the felt appears to become stiffer the more it compresses, so that a larger force must be applied to produce the same increase in compression. In this case, the wool felt used for piano hammers acts as a nonlinear hardening spring. Measurements (reported in the literature) of applied force versus felt compression for many different brands of piano hammers show that the nonlinear relationship between applied force and felt compression appears to be of the form F = K x p ,
where K is a generalized stiffness of the hammer, and the exponent p describes how the stiffness changes with applied force. The greater the value of p the greater the range of hammer stiffness for a given range of force. This exponent p is called the effective nonlinearity exponent
However, when a steadily increasing force is applied to a wool felt pad, the resulting compression does not obey the linear relationship F = k x. Instead, one finds that the felt appears to become stiffer the more it compresses, so that a larger force must be applied to produce the same increase in compression. In this case, the wool felt used for piano hammers acts as a nonlinear hardening spring. Measurements (reported in the literature) of applied force versus felt compression for many different brands of piano hammers show that the nonlinear relationship between applied force and felt compression appears to be of the form F = K x p ,
where K is a generalized stiffness of the hammer, and the exponent p describes how the stiffness changes with applied force. The greater the value of p the greater the range of hammer stiffness for a given range of force. This exponent p is called the effective nonlinearity exponent
 When a force F is applied to the felt of a piano hammer, the felt is compressed. The distance by which the felt is compressed depends on the amount of applied force. If the piano hammer felt behaved like a linear spring then the relationship between applied force F and compression x would be a linear relationship F = k x, where k is a constant depending on the properties of the spring. This linear relationship is known as Hooke's law.
When a force F is applied to the felt of a piano hammer, the felt is compressed. The distance by which the felt is compressed depends on the amount of applied force. If the piano hammer felt behaved like a linear spring then the relationship between applied force F and compression x would be a linear relationship F = k x, where k is a constant depending on the properties of the spring. This linear relationship is known as Hooke's law.

 While static measurements of the effective nonlinearity exponent p from force-compression curves are useful, they cannot completely describe what happens during an impact as the hammer strikes the string. In a dynamic measurement, a piano hammer strikes a piece(s) of piano wire attached to the face of a force transducer, securely mounted in a drill press. The force transducer measures the time-history (force versus time) of the hammer impact. Dynamic stiffness can be extracted from this force-time curve as will be shown below. Others (Boutillon, Yanagisawa and Nakamura, Askenfelt and Jansson) also measured the compression dynamically using gap sensors.
While static measurements of the effective nonlinearity exponent p from force-compression curves are useful, they cannot completely describe what happens during an impact as the hammer strikes the string. In a dynamic measurement, a piano hammer strikes a piece(s) of piano wire attached to the face of a force transducer, securely mounted in a drill press. The force transducer measures the time-history (force versus time) of the hammer impact. Dynamic stiffness can be extracted from this force-time curve as will be shown below. Others (Boutillon, Yanagisawa and Nakamura, Askenfelt and Jansson) also measured the compression dynamically using gap sensors.





 Hall and Askenfelt have developed a method of extracting the effective nonlinearity exponent p from a force-time curve measured from a hammer impact. They attempted to fit the upper portion of the force-time curve with a half-cycle sine curve. An effective stiffness may then be determined from SE = m π2 / τe, where m is the hammer mass, and τe is the effective time duration of the impact. τe is determined by measuring the halfwidth (pulse width at ½ peak) of the force-time curve (see figure at right).
Hall and Askenfelt have developed a method of extracting the effective nonlinearity exponent p from a force-time curve measured from a hammer impact. They attempted to fit the upper portion of the force-time curve with a half-cycle sine curve. An effective stiffness may then be determined from SE = m π2 / τe, where m is the hammer mass, and τe is the effective time duration of the impact. τe is determined by measuring the halfwidth (pulse width at ½ peak) of the force-time curve (see figure at right).

